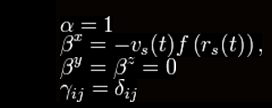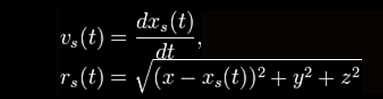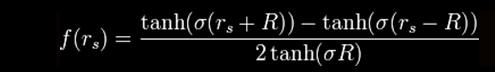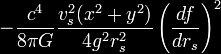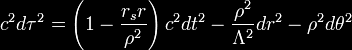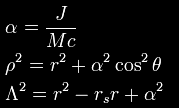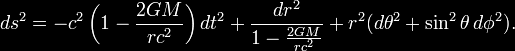by Dr. David Lewis Anderson

2008

from AndersonInstitute Website

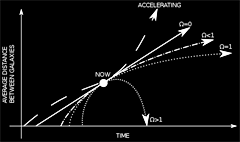
The ability to control time in both a forward and backwards direction is possible within the laws of our mathematics and physics. The chart below (click for larger view) compares ten different technologies an methods.

Key characteristics are identified for each and described below.

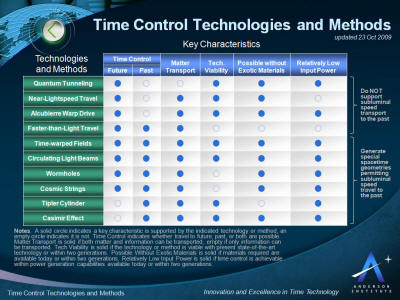
Under each key characteristic is a column with either a solid or empty circle.

A solid circle indicates a key characteristic is supported by the indicated technology or method, an empty circle indicates it is not.

• "Time Control" indicates whether travel to future, past, or both are possible.

• "Matter Transport" is solid if both matter and information can be transported, empty if only information can be transported.

• "Tech Viability" is solid if the technology or method is viable with present state-of-the-art technology or within two generations.

• "Possible Without Exotic Materials" is solid if materials required are available today or within two generations.

• "Relatively Low Input Power" is solid if time control is achievable within power generation capabilities available today or within two generations.

The time control technologies and methods above include the following:

Contents

1. Quantum Tunneling

2. Near-Lightspeed

3. Alcubierre Warp Drive

4. Faster-than-Light

5. Time-warped Field

6. Circulating Light Beams

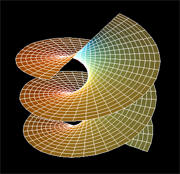
7. Wormholes

8. Cosmic Strings

9. Tipler Cylinder

10. Casimir Effect

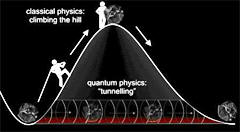
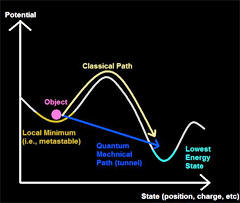
Quantum Tunneling

Quantum Tunneling is an evanescent wave coupling effect that occurs in quantum mechanics.

The correct wavelength combined with the proper tunneling barrier makes it possible to pass signals faster than light, backwards in time.

In the diagram above light pulses consisting of waves of various frequencies are shot toward a 10 centimeter chamber containing cesium vapor.

All information about the incoming pulse is contained in the leading edge of its waves. This information is all the cesium atoms need to replicate the pulse and send it out the other side.

At the same time it is believed an opposite wave rebounds inside the chamber cancelling out the main part of the incoming pulse as it enters the chamber. By this time the new pulse, moving faster than the speed of light, has traveled about 60 feet beyond the chamber. Essentially the pulse has left the chamber before it finished entering, traveling backwards in time.

The key characteristics of the application of quantum tunneling for time control and time travel are presented in the picture below.

This is followed by more detail describing the phenomenon below.

Wave-mechanical tunneling (also called quantum-mechanical tunneling, quantum tunneling, and the tunnel effect) is an evanescent wave coupling effect that occurs in the context of quantum mechanics because the behavior of particles is governed by Schrödinger's wave-equation.

All wave equations exhibit evanescent wave coupling effects if the conditions are right. Wave coupling effects mathematically equivalent to those called "tunneling" in quantum mechanics can occur with Maxwell's wave-equation (both with light and with microwaves), and with the common non-dispersive wave-equation often applied (for example) to waves on strings and to acoustics.

For these effects to occur there must be a situation where a thin region of "medium type 2" is sandwiched between two regions of "medium type 1", and the properties of these media have to be such that the wave equation has "traveling-wave" solutions in medium type 1, but "real exponential solutions" (rising and falling) in medium type 2.

In optics, medium type 1 might be glass, medium type 2 might be vacuum. In quantum mechanics, in connection with motion of a particle, medium type 1 is a region of space where the particle total energy is greater than its potential energy, medium type 2 is a region of space (known as the "barrier") where the particle total energy is less than its potential energy.

If conditions are right, amplitude from a traveling wave, incident on medium type 2 from medium type 1, can "leak through" medium type 2 and emerge as a traveling wave in the second region of medium type 1 on the far side. If the second region of medium type 1 is not present, then the traveling wave incident on medium type 2 is totally reflected, although it does penetrate into medium type 2 to some extent.

Depending on the wave equation being used, the leaked amplitude is interpreted physically as traveling energy or as a traveling particle, and, numerically, the ratio of the square of the leaked amplitude to the square of the incident amplitude gives the proportion of incident energy transmitted out the far side, or (in the case of the Schrödinger equation) the probability that the particle "tunnels" through the barrier.
 

Quantum Tunneling Introduction

Quantum Tunneling

The scale on which these "tunneling-like phenomena" occur depends on the wavelength of the traveling wave.

For electrons the thickness of "medium type 2" (called in this context "the tunneling barrier") is typically a few nanometers; for alpha-particles tunneling out of a nucleus the thickness is very much less; for the analogous phenomenon involving light the thickness is very much greater.

With Schrödinger's wave-equation, the characteristic that defines the two media discussed above is the kinetic energy of the particle if it is considered as an object that could be located at a point.

In medium type 1 the kinetic energy would be positive, in medium type 2 the kinetic energy would be negative. There is no inconsistency in this, because particles cannot physically be located at a point: they are always spread out ("delocalized") to some extent, and the kinetic energy of the delocalized object is always positive.

What is true is that it is sometimes mathematically convenient to treat particles as behaving like points, particular in the context of Newton's Second Law and classical mechanics generally. In the past, people thought that the success of classical mechanics meant that particles could always and in all circumstances be treated as if they were located at points.

But there never was any convincing experimental evidence that this was true when very small objects and very small distances are involved, and we now know that this viewpoint was mistaken. However, because it is still traditional to teach students early in their careers that particles behave like points, it sometimes comes as a big surprise for people to discover that it is well established that traveling physical particles always physically obey a wave-equation (even when it is convenient to use the mathematics of moving points).

Clearly, a hypothetical classical point particle analyzed according to Newton's Laws could not enter a region where its kinetic energy would be negative. But, a real delocalized object, that obeys a wave-equation and always has positive kinetic energy, can leak through such a region if conditions are right.

An approach to tunneling that avoids mention of the concept of "negative kinetic energy" is set out below in the section on "Schrödinger equation tunneling basics".

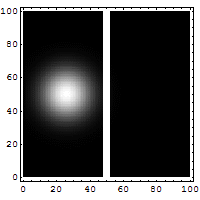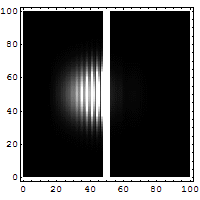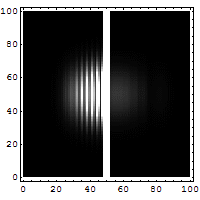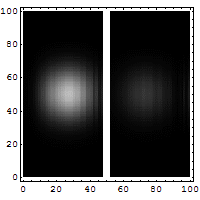
Reflection and tunneling of an electron
wave packet directed at a potential barrier.
The bright spot moving to the left is the
reflected part of the wave packet. A very
dim spot can be seen moving to the right
of the barrier. This is the small fraction of
the wave packet that tunnels through the
classically forbidden barrier. Also notice
the interference fringes between the
incoming and reflected waves.
 

An electron approaching a barrier has to be represented as a wave-train.

This wave-train can sometimes be quite long – electrons in some materials can be 10 to 20 nm long. This makes animations difficult. If it were legitimate to represent the electron by a short wave-train, then tunneling could be represented as in the animation alongside.

It is sometimes said that tunneling occurs only in quantum mechanics. Unfortunately, this statement is a bit of linguistic conjuring trick. As indicated above, "tunneling-type" evanescent-wave phenomena occur in other contexts too. But, until recently, it has only been in quantum mechanics that evanescent wave coupling has been called "tunneling". (However, there is an increasing tendency to use the label "tunneling" in other contexts too, and the names "photon tunneling" and "acoustic tunneling" are now used in the research literature.)

With regards to the mathematics of tunneling, a special problem arises. For simple tunneling-barrier models, such as the rectangular barrier, the Schrödinger equation can be solved exactly to give the value of the tunneling probability (sometimes called the "transmission coefficient").

Calculations of this kind make the general physical nature of tunneling clear.

One would also like to be able to calculate exact tunneling probabilities for barrier models that are physically more realistic. However, when appropriate mathematical descriptions of barriers are put into the Schrödinger equation, then the result is an awkward non-linear differential equation. Usually, the equation is of a type where it is known to be mathematically impossible in principle to solve the equation exactly in terms of the usual functions of mathematical physics, or in any other simple way.

Mathematicians and mathematical physicists have been working on this problem since at least 1813, and have been able to develop special methods for solving equations of this kind approximately. In physics these are known as "semi-classical" or "quasi-classical" methods. A common semi-classical method is the so-called WKB approximation (also known as the "JWKB approximation").

The first known attempt to use such methods to solve a tunneling problem in physics was made in 1928, in the context of field electron emission.

It is sometimes considered that the first people to get the mathematics of applying this kind of approximation to tunneling fully correct (and to give reasonable mathematical proof that they had done so) were N. Fröman and P.O. Fröman, in 1965. Their complex ideas have not yet made it into theoretical-physics textbooks, which tend to give simpler (but slightly more approximate) versions of the theory.

An outline of one particular semi-classical method is given below.

Three notes may be helpful. In general, students taking physics courses in quantum mechanics are presented with problems (such as the quantum mechanics of the hydrogen atom) for which exact mathematical solutions to the Schrödinger equation exist.

Tunneling through a realistic barrier is a reasonably basic physical phenomenon. So it is sometimes the first problem that students encounter where it is mathematically impossible in principle to solve the Schrödinger equation exactly in any simple way. Thus, it may also be the first occasion on which they encounter the "semi-classical-method" mathematics needed to solve the Schrödinger equation approximately for such problems.

Not surprisingly, this mathematics is likely to be unfamiliar, and may feel "odd". Unfortunately, it also comes in several different variants, which doesn't help.

Also, some accounts of tunneling seem to be written from a philosophical viewpoint that a particle is "really" point-like, and just has wave-like behavior. There is very little experimental evidence to support this viewpoint. A preferable philosophical viewpoint is that the particle is "really" delocalized and wave-like, and always exhibits wave-like behavior, but that in some circumstances it is convenient to use the mathematics of moving points to describe its motion. This second viewpoint is used in this section.

The precise nature of this wave-like behavior is, however, a much deeper matter, beyond the scope of this article on tunneling.

Although the phenomenon under discussion here is usually called "quantum tunneling" or "quantum-mechanical tunneling", it is the wave-like aspects of particle behavior that are important in tunneling theory, rather than effects relating to the quantization of the particle's energy states.

For this reason, some writers prefer to call the phenomenon "wave-mechanical tunneling.

History

George Gamow

By 1928, George Gamow had solved the theory of the alpha decay of a nucleus via tunneling.

Classically, the particle is confined to the nucleus because of the high energy requirement to escape the very strong potential. Under this system, it takes an enormous amount of energy to pull apart the nucleus. In quantum mechanics, however, there is a probability the particle can tunnel through the potential and escape. Gamow solved a model potential for the nucleus and derived a relationship between the half-life of the particle and the energy of the emission.

Alpha decay via tunneling was also solved concurrently by Ronald Gurney and Edward Condon. Shortly thereafter, both groups considered whether particles could also tunnel into the nucleus.

After attending a seminar by Gamow, Max Born recognized the generality of quantum-mechanical tunneling. He realized that the tunneling phenomenon was not restricted to nuclear physics, but was a general result of quantum mechanics that applies to many different systems.

Today the theory of tunneling is even applied to the early cosmology of the universe.

Quantum tunneling was later applied to other situations, such as the cold emission of electrons, and perhaps most importantly semiconductor and superconductor physics.

Phenomena such as field emission, important to flash memory, are explained by quantum tunneling. Tunneling is a source of major current leakage in Very-large-scale integration (VLSI) electronics, and results in the substantial power drain and heating effects that plague high-speed and mobile technology.

Another major application is in electron-tunneling microscopes which can resolve objects that are too small to see using conventional microscopes. Electron tunneling microscopes overcome the limiting effects of conventional microscopes (optical aberrations, wavelength limitations) by scanning the surface of an object with tunneling electrons.

Quantum tunneling has been shown to be a mechanism used by enzymes to enhance reaction rates. It has been demonstrated that enzymes use tunneling to transfer both electrons and nuclei such as hydrogen and deuterium.

It has even been shown, in the enzyme glucose oxidase, that oxygen nuclei can tunnel under physiological conditions.

Back to Contents




Near-Lightspeed Travel

Near-Lightspeed Travel has the ability to significantly dilate time, sending an accelerating traveler rapidly forward in time relative to those left behind before her travel.

The closer to the speed of light, the further into the future the travel.

The key characteristics of the application of near-lightspeed travel for time control and time travel are presented in the picture below.

This is followed by more detail describing the effect below.

Back to Contents




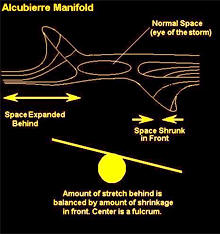
Alcubierre Warp Drive

An Alcubierre Warp Drive stretches spacetime in a wave causing the fabric of space ahead of a spacecraft to contract and the space behind it to expand.

The ship can ride the wave to accelerate to high speeds and time travel.

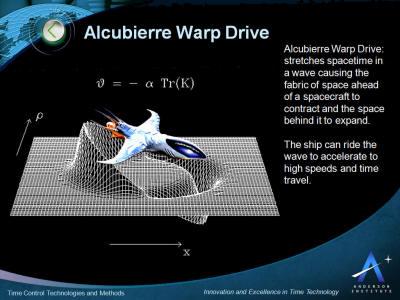
The Alcubierre drive, also known as the Alcubierre metric or Warp Drive, is a mathematical model of a spacetime exhibiting features reminiscent of the fictional "warp drive" from Star Trek, which can travel "faster than light" (although not in a local sense - see below).

The key characteristics of the application of Alcubierre warp drives for time control and time travel are presented in the picture below.

This is followed by more detail describing the effect below.

Alcubierre Warp Drive Description

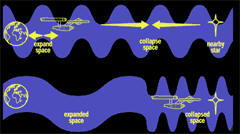
In 1994, the Mexican physicist Miguel Alcubierre proposed a method of stretching space in a wave which would in theory cause the fabric of space ahead of a spacecraft to contract and the space behind it to expand.

The ship would ride this wave inside a region known as a warp bubble of flat space. Since the ship is not moving within this bubble, but carried along as the region itself moves, conventional relativistic effects such as time dilation do not apply in the way they would in the case of a ship moving at high velocity through flat spacetime.

Also, this method of travel does not actually involve moving faster than light in a local sense, since a light beam within the bubble would still always move faster than the ship; it is only "faster than light" in the sense that, thanks to the contraction of the space in front of it, the ship could reach its destination faster than a light beam restricted to travelling outside the warp bubble.

Thus, the Alcubierre drive does not contradict the conventional claim that relativity forbids a slower-than-light object to accelerate to faster-than-light speeds.
 

Alcubierre Metric

The Alcubierre Metric defines the so-called warp drive spacetime.

This is a Lorentzian manifold which, if interpreted in the context of general relativity, exhibits features reminiscent of the warp drive from Star Trek: a warp bubble appears in previously flat spacetime and moves off at effectively superluminal speed. Inhabitants of the bubble feel no inertial effects. The object(s) within the bubble are not moving (locally) faster than light, instead, the space around them shifts so that the object(s) arrives at its destination faster than light would in normal space.

Alcubierre chose a specific form for the function f, but other choices give a simpler spacetime exhibiting the desired "warp drive" effects more clearly and simply.
 

Mathematics of the Alcubierre drive
Using the 3+1 formalism of general relativity, the spacetime is described by a foliation of space-like hypersurfaces of constant coordinate time t. The general form of the Alcubierre metric is:

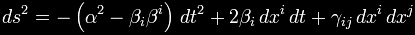
where α is the lapse function that gives the interval of proper time between nearby hypersurfaces, βI is the shift vector that relates the spatial coordinate systems on different hypersurfaces and γij is a positive definite metric on each of the hypersurfaces.

The particular form that Alcubierre studied is defined by:

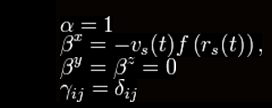
...where,

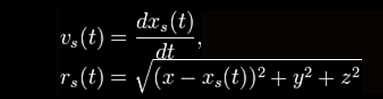
...and,

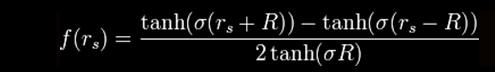
...with R > 0 and σ > 0 arbitrary parameters. Alcubierre's specific form of the metric can thus be written;

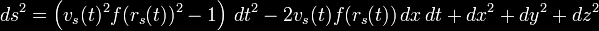
With this particular form of the metric, it can be shown that the energy density measured by observers whose 4-velocity is normal to the hypersurfaces is given by

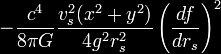
where g is the determinant of the metric tensor. Thus, as the energy density is negative, one needs exotic matter to travel faster than the speed of light.

The existence of exotic matter is not theoretically ruled out, the Casimir effect and the accelerating universe both lending support to the proposed existence of such matter.

However, generating enough exotic matter and sustaining it to perform feats such as faster-than-light travel (and also to keep open the 'throat' of a wormhole) is thought to be impractical.

Low has argued that within the context of general relativity, it is impossible to construct a warp drive in the absence of exotic matter.

It is generally believed that a consistent theory of quantum gravity will resolve such issues once and for all.
 

Physics of the Alcubierre drive

For those familiar with the effects of special relativity, such as Lorentz contraction and time dilation, the Alcubierre metric has some apparently peculiar aspects.

In particular, Alcubierre has shown that even when the ship is accelerating, it travels on a free-fall geodesic. In other words, a ship using the warp to accelerate and decelerate is always in free fall, and the crew would experience no accelerational g-forces.

Enormous tidal forces would be present near the edges of the flat-space volume because of the large space curvature there, but by suitable specification of the metric, these would be made very small within the volume occupied by the ship.

The original warp drive metric, and simple variants of it, happen to have the ADM form which is often used in discussing the initial value formulation of general relativity. This may explain the widespread misconception that this spacetime is a solution of the field equation of general relativity. Metrics in ADM form are adapted to a certain family of inertial observers, but these observers are not really physically distinguished from other such families.

Alcubierre interpreted his "warp bubble" in terms of a contraction of "space" ahead of the bubble and an expansion behind. But this interpretation might be misleading, since the contraction and expansion actually refers to the relative motion of nearby members of the family of ADM observers.

In general relativity, one often first specifies a plausible distribution of matter and energy, and then finds the geometry of the spacetime associated with it; but it is also possible to run the Einstein field equations in the other direction, first specifying a metric and then finding the energy-momentum tensor associated with it, and this is what Alcubierre did in building his metric.

This practice means that the solution can violate various energy conditions and require exotic matter.

The need for exotic matter leads to questions about whether it is actually possible to find a way to distribute the matter in an initial spacetime which lacks a "warp bubble" in such a way that the bubble will be created at a later time. Yet another problem is that, according to Serguei Krasnikov, it would be impossible to generate the bubble without being able to force the exotic matter to move at locally FTL speeds, which would require the existence of tachyons.

Some methods have been suggested which would avoid the problem of tachyonic motion, but would probably generate a naked singularity at the front of the bubble.
 

Difficulties

Significant problems with the metric of this form stem from the fact that all known warp drive spacetimes violate various energy conditions.

It is true that certain experimentally verified quantum phenomena, such as the Casimir effect, when described in the context of the quantum field theories, lead to stress-energy tensors which also violate the energy conditions and so one might hope that Alcubierre type warp drives could perhaps be physically realized by clever engineering taking advantage of such quantum effects.

However, if certain quantum inequalities conjectured by Ford and Roman hold, then the energy requirements for some warp drives may be absurdly gigantic, e.g. the energy -1067gram equivalent might be required to transport a small spaceship across the Milky Way galaxy. This is orders of magnitude greater than the mass of the universe.

Counterarguments to these apparent problems have been offered, but not everyone is convinced they can be overcome.

Chris Van Den Broeck, in 1999, has tried to address the potential issues.

By contracting the 3+1 dimensional surface area of the 'bubble' being transported by the drive, while at the same time expanding the 3 dimensional volume contained inside, Van Den Broeck was able to reduce the total energy needed to transport small atoms to less than 3 solar masses. Later, by slightly modifying the Van Den Broeck metric, Krasnikov reduced the necessary total amount of negative energy to a few milligrams.

Krasnikov proposed that, if tachyonic matter could not be found or used, then a solution might be to arrange for masses along the path of the vessel to be set in motion in such a way that the required field was produced. But in this case the Alcubierre Drive vessel is not able to go dashing around the galaxy at will.

It is only able to travel routes which, like a railroad, have first been equipped with the necessary infrastructure.

Miguel Alcubierre

The pilot inside the bubble is causally disconnected with its walls and cannot carry out any action outside the bubble.

However, it is necessary to place devices along the route in advance, and since the pilot cannot do this while "in transit", the bubble cannot be used for the first trip to a distant star. In other words, to travel to Vega (which is 26 light-years from the Earth) one first has to arrange everything so that the bubble moving toward Vega with a superluminal velocity would appear and these arrangements will always take more than 26 years.

Coule has argued that schemes such as the one proposed by Alcubierre are not feasible because the matter to be placed on the road beforehand has to be placed at superluminal speed. Thus, according to Coule, an Alcubierre Drive is required in order to build an Alcubierre Drive. Since none have been proven to exist already then the drive is impossible to construct, even if the metric is physically meaningful.

Coule argues that an analogous objection will apply to any proposed method of constructing an Alcubierre Drive.

Back to Contents


 

Faster-than-Light Travel

Faster-than-Light Travel is an interesting and controversial subject. According to special relativity anything that could travel faster-than-light would move backward in time.

As the same time, special relativity states that this would require infinite energy.

Faster-than-light (also superluminal or FTL) communications and travel refer to the propagation of information or matter faster than the speed of light.

Under the special theory of relativity, a particle (that has mass) with subluminal velocity needs infinite energy to accelerate to the speed of light, although special relativity does not forbid the existence of particles that travel faster than light at all times.

On the other hand, what some physicists refer to as "apparent" or "effective" FTL is the hypothesis that unusually distorted regions of spacetime might permit matter to reach distant locations faster than what it would take light in the "normal" route (though still moving subluminally through the distorted region).

Apparent FTL is not excluded by general relativity. Examples of apparent FTL proposals are the Alcubierre drive and the traversable wormhole, although the physical plausibility of these solutions is uncertain.

The key characteristics of the application of faster-than-light travel for time control and time travel are presented in the picture below.

This is followed by more detail describing the effect below.

Outside of mainstream physics, others have speculated on mechanisms that might allow FTL travel to be achieved, often relying on new conjectures of physics of their own invention, but their ideas have not gained significant acceptance in the physics research community.

Fictional depictions of superluminal travel and the mechanisms of achieving it are also a staple of the science fiction genre.

Travel

In the context of this article, FTL is transmitting information or matter faster than c, a constant equal to the speed of light in a vacuum, 299,792,458 meters per second, or about 186,282 miles per second.

This is not quite the same as traveling faster than light, since:

• Some processes propagate faster than c, but cannot carry information.
   

• Light travels at speed c/n when not in a vacuum but traveling through a medium with refractive index = n (causing refraction), and in some materials other particles can travel faster than c/n (but still slower than c), leading to Cherenkov radiation.

Neither of these phenomena violates special relativity or creates problems with causality, and thus neither qualifies as FTL as described here.

 

Possibility

Faster-than-light communication is, by Einstein's theory of relativity, equivalent to time travel.

According to Einstein's theory of special relativity, what we measure as the speed of light in a vacuum is actually the fundamental physical constant c. This means that all observers, regardless of their relative velocity, will always measure zero-mass particles such as photons traveling at c in a vacuum. This result means that measurements of time and velocity in different frames are no longer related simply by constant shifts, but are instead related by Poincaré transformations.

These transformations have important implications:

• The relativistic momentum of a massive particle would increase with speed in such a way that at the speed of light an object would have infinite momentum.
   

• To accelerate an object of non-zero rest mass to c would require infinite time with any finite acceleration, or infinite acceleration for a finite amount of time.
   

• Either way, such acceleration requires infinite energy. Going beyond the speed of light in a homogeneous space would hence require more than infinite energy, which is not generally considered to be a sensible notion.
   

• Some observers with sub-light relative motion will disagree about which occurs first of any two events that are separated by a space-like interval. In other words, any travel that is faster-than-light will be seen as traveling backwards in time in some other, equally valid, frames of reference, or need to assume the speculative hypothesis of possible Lorentz violations at a presently unobserved scale (for instance the Planck scale).

   

  Therefore any theory which permits "true" FTL also has to cope with time travel and all its associated paradoxes, or else to assume the Lorentz invariance to be a symmetry of thermodynamical statistical nature (hence a symmetry broken at some presently unobserved scale).
   

• While Special and general relativity do not allow superluminal speeds locally, non-local means may be possible, which means moving with space rather than moving through space.

Justifications

Despite the established conclusion that relativity precludes FTL travel, some have proposed ways to justify FTL behavior:
 

Radically Curve Spacetime Using Slip String Drive

There is one way that doesn't violate Relativity. Andrew L. Bender's Slip String Drive.

Bender proposes traveling by completely isolating a region of spacetime from the rest of our universe using Einstein's gravity waves. These compression waves of spacetime are generated by a ship, which emits them from its hull in all directions until it is completely isolated from the rest of our universe. Then, by emitting more gravity waves behind the ship, it stretches out its isolated bubble into an egg-shape, causing external spacetime to squeeze in on the bubble unevenly, propelling the craft forward at speeds no longer limited by relativity.

Time passes normally within the isolated region, eliminating the possibility of paradox or time travel.
 

Ignore special relativity

This option is popular particularly in science fiction. However, empirical and theoretical evidence strongly supports Einstein's theory of special relativity as the correct description of high-speed motion, which generalizes the more familiar Galilean relativity, which is actually an approximation at conventional (much less than c) speeds.

Similarly, general relativity is an overwhelmingly supported and experimentally verified theory of gravitation, except in the regime of very high energy densities over very short distances, where an as-yet-undeveloped theory of quantum gravity is necessary. Special relativity, however, is incorporated easily into quantum field theories.

Therefore, even in the broader contexts of general relativity and quantum mechanics, conventional acceleration from subluminal to superluminal speeds is not possible.
 

Faster light (Casimir vacuum and quantum tunneling)

Einstein's equations of special relativity postulate that the speed of light in a vacuum is invariant in inertial frames.

That is, it will be the same from any frame of reference moving at a constant speed. The equations do not specify any particular value for the speed of the light, which is an experimentally determined quantity for a fixed unit of length.

Since 1983, the unit of length (the meter) has been defined using the speed of light.

Casimir Vacuum Force

The experimental determination has been made in vacuum.

However, the vacuum we know is not the only possible vacuum which can exist. The vacuum has energy associated with it, called the vacuum energy. This vacuum energy can perhaps be changed in certain cases. When vacuum energy is lowered, light itself has been predicted to go faster than the standard value 'c'. This is known as the Scharnhorst effect.

Such a vacuum can be produced by bringing two perfectly smooth metal plates together at near atomic diameter spacing. It is called a Casimir vacuum. Calculations imply that light will go faster in such a vacuum by a minuscule amount: a photon traveling between two plates that are 1 micrometer apart would increase the photon's speed by only about one part in 1036.

Accordingly there has as yet been no experimental verification of the prediction. A recent analysis argued that the Scharnhorst effect cannot be used to send information backwards in time with a single set of plates since the plates' rest frame would define a "preferred frame" for FTL signaling.

However, with multiple pairs of plates in motion relative to one another the authors noted that they had no arguments that could "guarantee the total absence of causality violations", and invoked Hawking's speculative chronology protection conjecture which suggests that feedback loops of virtual particles would create "uncontrollable singularities in the renormalized quantum stress-energy" on the boundary of any potential time machine, and thus would require a theory of quantum gravity to fully analyze.

Other authors argue that Scharnhorst's original analysis which seemed to show the possibility of faster-than-c signals involved approximations which may be incorrect, so that it is not clear whether this effect could actually increase signal speed at all.

The physicists Günter Nimtz and Alfons Stahlhofen, of the University of Koblenz, claim to have violated relativity experimentally by transmitting photons faster than the speed of light. They say they have conducted an experiment in which microwave photons - relatively low energy packets of light - travelled "instantaneously" between a pair of prisms that had been moved up to 3 ft apart, using a phenomenon known as quantum tunneling.

Nimtz told New Scientist magazine:

"For the time being, this is the only violation of special relativity that I know of."

However, other physicists say that this phenomenon does not allow information to be transmitted faster than light.

Aephraim Steinberg, a quantum optics expert at the University of Toronto, Canada, uses the analogy of a train traveling from Chicago to New York, but dropping off train cars at each station along the way, so that the center of the train moves forward at each stop; in this way, the speed of the center of the train exceeds the speed of any of the individual cars.
 

Give up causality

Another approach is to accept special relativity, but to posit that mechanisms allowed by general relativity (e.g., wormholes) will allow traveling between two points without going through the intervening space.

While this gets around the infinite acceleration problem, it still would lead to closed timelike curves (i.e., time travel) and causality violations. Causality is not required by special or general relativity, but is nonetheless generally considered a basic property of the universe that cannot be sensibly dispensed with. Because of this, most physicists expect (or perhaps hope) that quantum gravity effects will preclude this option.

An alternative is to conjecture that, while time travel is possible, it never leads to paradoxes; this is the Novikov self-consistency principle.

An important point to note is that in general relativity it is possible for objects to be moving apart faster than light because of the expansion of the universe, in some reasonable choice of cosmological coordinates.

This is understood to be due to the expansion of the space between the objects, and general relativity still reduces to special relativity in a "local" sense, meaning that two objects passing each other in a small local region of spacetime cannot have a relative velocity greater than c, and will move more slowly than a light beam passing through the region.
 

Give up (absolute) relativity

Because of the strong empirical support for special relativity, any modifications to it must necessarily be quite subtle and difficult to measure.

The best-known attempt is doubly-special relativity, which posits that the Planck length is also the same in all reference frames, and is associated with the work of Giovanni Amelino-Camelia and João Magueijo.

One consequence of this theory is a variable speed of light, where photon speed would vary with energy, and some zero-mass particles might possibly travel faster than c. However, even if this theory is accurate, it is still very unclear whether it would allow information to be communicated, and appears not in any case to allow massive particles to exceed c.

There are speculative theories that claim inertia is produced by the combined mass of the universe (e.g., Mach's principle), which implies that the rest frame of the universe might be preferred by conventional measurements of natural law.

If confirmed, this would imply special relativity is an approximation to a more general theory, but since the relevant comparison would (by definition) be outside the observable universe, it is difficult to imagine (much less construct) experiments to test this hypothesis.
 

Non-physical realms

A very popular option in space opera is to assume the existence of some other realm (typically called hyperspace, subspace, or slipspace) which is accessible from this universe, in which the laws of relativity are usually distorted, bent, or nonexistent, facilitating rapid transport between distant points in this universe, sometimes with acceleration differences - that is, not requiring as much energy or thrust to go faster.

To accomplish rapid transport between points in hyperspace/subspace, special relativity is often assumed not to apply in this other realm, or that the speed of light is higher. Another solution is to posit that distant points in the mundane universe correspond to points that are close together in hyperspace.

This method of faster-than-light travel does not correspond to anything seriously proposed by mainstream science.
 

Space-time distortion

Although the theory of special relativity forbids objects to have a relative velocity greater than light speed, and general relativity reduces to special relativity in a local sense (in small regions of spacetime where curvature is negligible), general relativity does allow the space between distant objects to expand in such a way that they have a "recession velocity" which exceeds the speed of light, and it is thought that galaxies which are at a distance of more than about 14 billion light years from us today have a recession velocity which is faster than light.

Miguel Alcubierre theorized that it would be possible to create an Alcubierre drive, in which a ship would be enclosed in a "warp bubble" where the space at the front of the bubble is rapidly contracting and the space at the back is rapidly expanding, with the result that the bubble can reach a distant destination much faster than a light beam moving outside the bubble, but without objects inside the bubble locally traveling faster than light.

However, several objections raised against the Alcubierre drive appear to rule out the possibility of actually using it in any practical fashion. Another possibility predicted by general relativity is the traversable wormhole, which could create a shortcut between arbitrarily distant points in space.

As with the Alcubierre drive, travelers moving through the wormhole would not locally move faster than light which travels through the wormhole alongside them, but they would be able to reach their destination (and return to their starting location) faster than light traveling outside the wormhole.

Dr. Gerald Cleaver, associate professor of physics at Baylor University, and Richard Obousy, a Baylor graduate student, theorize that by manipulating the extra spatial dimensions of string theory around a spaceship with an extremely large amount of energy, it would create a “bubble” that could cause the ship to travel faster than the speed of light. To create this bubble, the physicists believe manipulating the 10th spatial dimension would alter the dark energy in three large spatial dimensions: height, width and length.

Cleaver said positive dark energy is currently responsible for speeding up the expansion rate of our universe as time moves on.
 

Heim theory

In 1977, a controversial paper on Heim theory theorized that it may be possible to travel faster than light by using magnetic fields to enter a higher-dimensional space, and the paper received some media attention in January 2006.

However, due to the many unproven assumptions in the paper, there have been few serious attempts to conduct further experiments.
 

Quantized space and time

As given by the Planck length, there is a minimum amount of 'space' that can exist in this universe (1.616×10−35 meters).

This limit can be used to determine a minimum time quantization of 5.391×10−44 seconds, which corresponds to a beam of light with a wavelength approaching the Planck length. This means that there is a physical limit to how much blue shift a beam of light can endure. According to general relativity there is no limit to this shift, and an infinitesimally small space can exist, but according to well accepted quantum theory these limits do exist.

This is precisely what happens towards the center of a black hole; the incoming light becomes blue shifted past the Planck length as it approaches the region of discontinuity within our universe. The argument is: if a black hole with finite mass can create such a discontinuity in the fabric of space and time, why would people be unable to do the same thing using a finite amount of energy and acceleration?

(According to general relativity, the space-time distortions caused by gravity are fundamentally identical to space-time distortions caused simply by accelerating your reference frame).
 

Tachyons

In special relativity, while it is impossible to accelerate an object to the speed of light, or for a massive object to move at the speed of light, it is not impossible for an object to exist which always moves faster than light.

The hypothetical elementary particles that have this property are called tachyons. Their existence has neither been proven nor disproven, but even so, attempts to quantize them show that they may not be used for faster-than-light communication.

Physicists sometimes regard the existence of mathematical structures similar to Tachyons arising from theoretical models and theories as signs of an inconsistency or that the theory needs further refining.
 

General relativity

General relativity was developed after special relativity to include concepts like gravity.

It maintains the principle that no object can accelerate to the speed of light in the reference frame of any coincident observer. However, it permits distortions in spacetime that allow an object to move faster than light from the point of view of a distant observer. One such distortion is the Alcubierre drive, which can be thought of as producing a ripple in spacetime that carries an object along with it.

Another possible system is the wormhole, which connects two distant locations as though by a shortcut. Both distortions would need to create a very strong curvature in a highly localized region of space-time and their gravity fields would be immense. To counteract the unstable nature, and prevent the distortions from collapsing under their own 'weight', one would need to introduce hypothetical exotic matter or negative energy.

General relativity also agrees that any technique for faster-than-light travel could also be used for time travel. This raises problems with causality. Many physicists believe that the above phenomena are in fact impossible, and that future theories of gravity will prohibit them.

One theory states that stable wormholes are possible, but that any attempt to use a network of wormholes to violate causality would result in their decay. In string theory Eric Gimon and Petr Hořava have argued that in a supersymmetric five-dimensional Gödel universe quantum corrections to general relativity effectively cut off regions of spacetimes with causality-violating closed timelike curves.

In particular, in the quantum theory a smeared supertube is present that cuts the spacetime in such a way that, although in the full spacetime a closed timelike curve passed through every point, no complete curves exist on the interior region bounded by the tube.
 

FTL phenomena

In these examples, certain influences may appear to travel faster than light, but they do not convey energy or information faster than light, so they do not violate special relativity.

Daily motion of the Heavens

 

 

 

 

For an earthbound observer objects in the sky complete one revolution around the earth in 1 day.

 

Alpha Centauri which is the nearest star outside the Solar system is about 4 light years away. On a geostationary view Alpha Centauri has a speed many times greater than "c" as the rim speed of an object moving in a circle is a product of the radius and angular speed. It is also possible on a geostatic view for objects such as comets to vary their speed from subluminal to superluminal and vice versa simply because the distance from the earth varies. Comets may have orbits which take them out to more than 1000 AU.

 

Circumference of a circle radius 1000 AU is greater than one light day. In other words, a comet at such a distance is superluminal in a geostatic frame.
 

 

Light spots and shadows
If a laser is swept across a distant object, the spot of light can easily be made to move at a speed greater than c. Similarly, a shadow projected onto a distant object can be made to move faster than c. In neither case does any matter or information travel faster than light.
 

 

Closing speeds
An observer may conclude that two objects are moving faster than the speed of light relative to each other, by adding their velocities according to the principle of Galilean relativity.

For example, two fast-moving particles approaching each other from opposite sides of a particle accelerator will appear to be moving at slightly less than twice the speed of light, relative to each other, from the point of view of an observer standing at rest relative to the accelerator.

 

This correctly reflects the rate at which the distance between the two particles is decreasing, from the observer's point of view and is called the closing speed. However, it is not the same as the velocity of one of the particles as would be measured by a hypothetical fast-moving observer traveling alongside the other particle. To obtain this, the calculation must be done according to the principle of special relativity.

 

If the two particles are moving at velocities v and -v, or expressed in units of c, β and − β, where

 

 

 

 

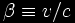
then this relative velocity (again in units of the speed of light c) is

 

 

 

 

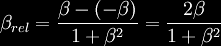
which is less than the speed of light.
 

Proper speeds
If a spaceship travels to a planet one light year (as measured in the Earth's rest frame) away from Earth at high speed, the time taken to reach that planet could be less than one year as measured by the traveler's clock (although it will always be more than one year as measured by a clock on Earth).

 

The value obtained by dividing the distance traveled, as determined in the Earth's frame, by the time taken, measured by the traveler's clock, is known as a proper speed or a proper velocity. There is no limit on the value of a proper speed as a proper speed does not represent a speed measured in a single inertial frame.

 

A light signal that left the Earth at the same time as the traveler would always get to the destination before the traveler.
 

 

Phase velocities above c

 

 

 

 

The phase velocity of an electromagnetic wave, when traveling through a medium, can routinely exceed c, the vacuum velocity of light.

 

For example, this occurs in most glasses at X-ray frequencies. However, the phase velocity of a wave corresponds to the propagation speed of a theoretical single-frequency (purely monochromatic) component of the wave at that frequency. Such a wave component must be infinite in extent and of constant amplitude (otherwise it is not truly monochromatic), and so cannot convey any information.

 

Thus a phase velocity above c does not imply the propagation of signals with a velocity above c.

 

Group velocities above c
The group velocity of a wave (e.g. a light beam) may also exceed c in some circumstances. In such cases, which typically at the same time involve rapid attenuation of the intensity, the maximum of the envelope of a pulse may travel with a velocity above c.

 

However, even this situation does not imply the propagation of signals with a velocity above c, even though one may be tempted to associate pulse maxima with signals. The latter association has been shown to be misleading, basically because the information on the arrival of a pulse can be obtained before the pulse maximum arrives.

 

For example, if some mechanism allows the full transmission of the leading part of a pulse while strongly attenuating the pulse maximum and everything behind, the pulse maximum is effectively shifted forward in time, while the information on the pulse does not come faster than without this effect.

 

Universal expansion

 

 

 

 

The expansion of the universe causes distant galaxies to recede from us faster than the speed of light, if commoving distance and cosmological time are used to calculate the speeds of these galaxies.

 

However, in general relativity, velocity is a local notion, so velocity calculated using commoving coordinates does not have any simple relation to velocity calculated locally. Rules that apply to relative velocities in special relativity, such as the rule that relative velocities cannot increase past the speed of light, do not apply to relative velocities in commoving coordinates, which are often described in terms of the "expansion of space" between galaxies.

 

This expansion rate is thought to have been at its peak during the inflationary epoch thought to have occurred in a tiny fraction of the second after the Big Bang (models suggest the period would have been from around 10-36 seconds after the Big Bang to around 10-33 seconds), when the universe may have rapidly expanded by a factor of around 1020 – 1030.

 

Astronomical observations
Apparent superluminal motion is observed in many radio galaxies, blazars, quasars and recently also in microquasars.

 

The effect was predicted before it was observed by Martin Rees and can be explained as an optical illusion caused by the object partly moving in the direction of the observer, when the speed calculations assume it does not.

 

The phenomenon does not contradict the theory of special relativity. Interestingly, corrected calculations show these objects have velocities close to the speed of light (relative to our reference frame). They are the first examples of large amounts of mass moving at close to the speed of light.

 

Earth-bound laboratories have only been able to accelerate small numbers of elementary particles to such speeds.

 

Quantum mechanics

 

 

 

 

Certain phenomena in quantum mechanics, such as quantum entanglement, appear to transmit information faster than light.

 

According to the No-communication theorem these phenomena do not allow true communication; they only let two observers in different locations see the same event simultaneously, without any way of controlling what either sees. Wavefunction collapse can be viewed as an epiphenomenon of quantum decoherence, which in turn is nothing more than an effect of the underlying local time evolution of the wavefunction of a system and all of its environment.

 

Since the underlying behavior doesn't violate local causality or allow FTL it follows that neither does the additional effect of wavefunction collapse, whether real or apparent.

The uncertainty principle implies that individual photons may travel for short distances at speeds somewhat faster (or slower) than c, even in a vacuum; this possibility must be taken into account when enumerating Feynman diagrams for a particle interaction.

 

To quote Richard Feynman:

…there is also an amplitude for light to go faster (or slower) than the conventional speed of light. You found out in the last lecture that light doesn't go only in straight lines; now, you find out that it doesn't go only at the speed of light! It may surprise you that there is an amplitude for a photon to go at speeds faster or slower than the conventional speed, c.
– Richard Feynman

However, macroscopically these fluctuations average out, so that photons do travel in straight lines over long (i.e. non-quantum) distances, and they do travel at the speed of light on average.

 

Therefore, this does not imply the possibility of superluminal information transmission.

There have been various reports in the popular press of experiments on faster-than-light transmission in optics - most often in the context of a kind of quantum tunneling phenomenon. Usually, such reports deal with a phase velocity or group velocity faster than the vacuum velocity of light. But, recall from above, that a superluminal phase velocity cannot be used for faster-than-light transmission of information.

 

There has sometimes been confusion concerning the latter point.

 

 

 

 

Quantum teleportation transmits quantum information at whatever speed is used to transmit the same amount of classical information, likely the speed of light.

 

This quantum information may theoretically be used in ways that classical information can not, such as in quantum computations involving quantum information only available to the recipient.

 

In science fiction, quantum teleportation is either used as a basis for teleportation of physical objects at the speed of light, presumably preserving some important aspect of the entanglement between the particles of the object, or else is misrepresented as allowing faster-than-light communication.

Say you have 4 pairs of entangled matter such that (x0,y0) are distinct from and won't affect (x1,y1), (x2,y2), etc. If y0 changes you know that x0 changed, the same being true for the other pairs. Right there you have a nibble's worth of information transfer any time x0, x1, x2, etc. are changed immediately altering y0, y1, and y2 respectively. Monitoring the y bits will immediately tell you when the entangled x bits are updated.
– SkewsMe.com

Hartman effect

 

 

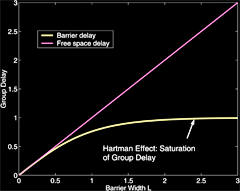
The Hartman Effect

 

The Hartman effect is the tunneling effect through a barrier where the tunneling time tends to a constant for large barriers.

 

This was first described by Thomas Hartman in 1962. This could, for instance, be the gap between two prisms. When the prisms are in contact, the light passes straight through, but when there is a gap, the light is refracted. There is a finite probability that the photon will tunnel across the gap rather than follow the refracted path. For large gaps between the prisms the tunneling time approaches a constant and thus the photons appear to have crossed with a superluminal speed.

However, an analysis by Herbert Winful from the University of Michigan suggests that the Hartman effect cannot actually be used to violate relativity by transmitting signals faster than c, because the tunneling time,

"should not be linked to a velocity since evanescent waves do not propagate".

Winful means by this that the photons crossing the barrier are virtual photons only existing in the interaction and could not be propagated into the outside world.

 

Casimir effect
In physics, the Casimir effect or Casimir-Polder force is a physical force exerted between separate objects due to resonance of vacuum energy in the intervening space between the objects.

 

This is sometimes described in terms of virtual particles interacting with the objects, due to the mathematical form of one possible way of calculating the strength of the effect. Because the strength of the force falls off rapidly with distance, it is only measurable when the distance between the objects is extremely small. Energy appears suddenly as if it came from the vacuum.

 

See Option B above for a discussion of whether or not this effect could actually be used to send signals faster than c or violate causality.

 

EPR Paradox

 

 

 

 

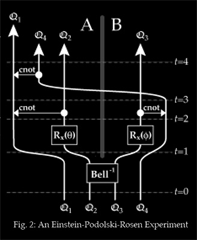
We can also quote the spectacular case of the thought experiment of Einstein, Podolski and Rosen (EPR paradox) which could be realized in experiments for the first time by Alain Aspect in 1981 and 1982 in the Aspect experiment.

 

In this case, the measurement of the state on one of the quantum systems of an entangled pair forces the other system to be measured in the complementary state. Thus functions quantum teleportation.

An experiment performed in 1997 by Nicolas Gisin at the University of Geneva has demonstrated nonlocal quantum correlations between particles separated by over 10 kilometers. But as noted earlier, the nonlocal correlations seen in entanglement cannot actually be used to transmit classical information faster than light, so that relativistic causality is preserved; see no-communication theorem for further information.

 

A 2008 quantum physics experiment also performed by Nicolas Gisin and his colleagues in Geneva, Switzerland has determined that the "speed" of the quantum non-local connection (what Einstein called spooky action at a distance) has a minimum lower bound of 10,000 times the speed of light.

 

Delayed choice quantum eraser

 

 

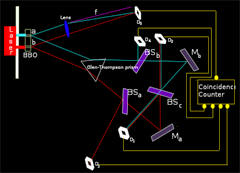
Delayed Choice Quantum Eraser

 

Delayed choice quantum eraser (an experiment of Marlan Scully) is a version of the EPR paradox in which the observation or not of interference after the passage of a photon through a double slit experiment depends on the conditions of observation of a second photon entangled with the first.

 

The characteristic of this experiment is that the observation of the second photon can take place at a later time than the observation of the first photon, which may give the impression that the measurement of the later photons "retroactively" determines whether the earlier photons show interference or not, although the interference pattern can only be seen by correlating the measurements of both members of every pair and so it can't be observed until both photons have been measured, ensuring that an experimenter watching only the photons going through the slit does not obtain information about the other photons in an FTL or backwards-in-time manner.

 

Variable speed of light
In conventional physics, the speed of light in a vacuum is assumed to be a constant. There exist theories which postulate that the speed of light is not a constant.

 

The interpretation of this statement is as follows.

 

 

Variable Speed of Light

 

 

The speed of light is a dimensional quantity and so, as has been emphasized in this context by João Magueijo, it cannot be measured.

 

Measurable quantities in physics are, without exception, dimensionless, although they are often constructed as ratios of dimensional quantities. For example, when you measure the height of a mountain you really measure the ratio of its height to the length of a meterstick. The conventional SI system of units is based on seven basic dimensional quantities, namely distance, mass, time, electric current, thermodynamic temperature, amount of substance, and luminous intensity.

 

These units are defined to be independent and so cannot be described in terms of each other. As an alternative to using a particular system of units, one can reduce all measurements to dimensionless quantities expressed in terms of ratios between the quantities being measured and various fundamental constants such as Newton's constant, the speed of light and Planck's constant; physicists can define at least 26 dimensionless constants which can be expressed in terms of these sorts of ratios and which are currently thought to be independent of one another.

 

By manipulating the basic dimensional constants one can also construct the Planck time, Planck length and Planck energy which make a good system of units for expressing dimensional measurements, known as Planck units.

Magueijo's proposal used a different set of units, a choice which he justifies with the claim that some equations will be simpler in these new units.

 

In the new units he fixes the fine structure constant, a quantity which some people, using units in which the speed of light is fixed, have claimed is time dependent. Thus in the system of units in which the fine structure constant is fixed, the observational claim is that the speed of light is time-dependent.

While it may be mathematically possible to construct such a system, it is not clear what additional explanatory power or physical insight such a system would provide, assuming that it does indeed accord with existing empirical data.

Back to Contents


 

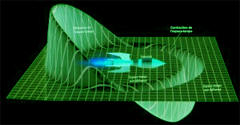
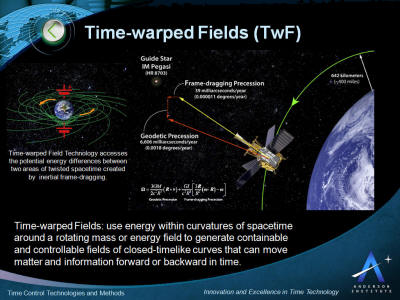
Time-warped Fields

Time-warped Fields use energy within curvatures of spacetime surrounding a rotating mass or energy field to generate containable and controllable fields of closed-timelike curves that can move matter and information forward or backward in time.

David Lewis Anderson, USAF
Officer and Scientist, founder of time-warped field theory.

As general relativity predicts, rotating bodies drag spacetime around themselves in a phenomenon referred to as frame-dragging.

This rotational frame-dragging effect is also known as the Lense-Thirring effect. The rotation of an object alters space and time, dragging a nearby object out of position compared to the predictions of Newtonian physics. The predicted effect is small - about one part in a few trillion.

However, as Dr. David Lewis Anderson proposed in 1987 with his announcement of time-warped field theory, the difference in potential energy between two different areas of twisted spacetime due to frame-dragging is significantly large. Even the smallest twist in spacetime contains enormous energy potential and can be used to create containable and controllable fields of close-timelike curves without the need for significant input power. This makes both forward and reverse time control possible within the limits of technology today.

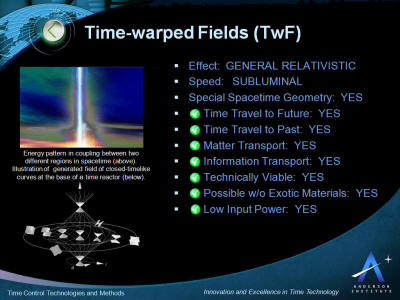
The key characteristics of the application of time-warped fields for time control and time travel are presented in the picture below.

This is followed by more detail describing the science below.

Frame Dragging Effect Basics

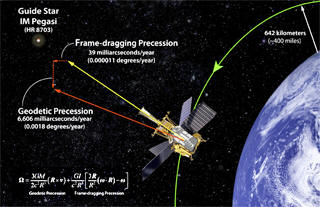
The Anderson Time Reactor operates by accessing the high energy
potential and effects, existing across two regions of twisted spacetime,
to create containable and controllable fields of closed-timelike curves.

Rotational frame-dragging appears in the general principle of relativity and similar theories in the vicinity of rotating massive objects.

Under this effect, the frame of reference in which a clock ticks the fastest is one which is rotating around the object as viewed by a distant observer. This also means that light traveling in the direction of rotation of the object will move around the object faster than light moving against the rotation as seen by a distant observer. It is now the best-known effect, partly thanks to the Gravity Probe B experiment.

Linear frame dragging is the similarly inevitable result of the general principle of relativity, applied to linear momentum. Although it arguably has equal theoretical legitimacy to the "rotational" effect, the difficulty of obtaining an experimental verification of the effect means that it receives much less discussion and is often omitted from articles on frame-dragging

Static mass increase is another effect.

The effect is an increase in inertia of a body when other masses are placed nearby. While not strictly a frame dragging effect, it is also derived from the same equation of general relativity. It is a tiny effect that is difficult to confirm experimentally.

Mathematical Derivation of Frame Dragging

Frame-dragging may be illustrated most readily using the Kerr metric, which describes the geometry of spacetime in the vicinity of a mass M rotating with angular momentum J

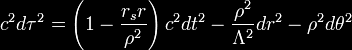
where rs is the Schwarzschild radius

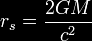
and where the following shorthand variables have been introduced for brevity

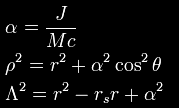
In the non-relativistic limit where M (or, equivalently, rs) goes to zero, the Kerr metric becomes the orthogonal metric for the oblate spheroidal coordinates

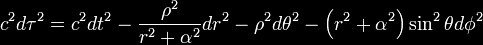
We may re-write the Kerr metric in the following form

This metric is equivalent to a co-rotating reference frame that is rotating with angular speed Ω that depends on both the radius r and the colatitude θ

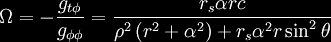
In the plane of the equator this simplifies to:

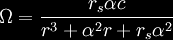
Thus, an inertial reference frame is entrained by the rotating central mass to participate in the latter's rotation; this is frame-dragging. Frame-dragging occurs about every rotating mass and at every radius r and colatitude θ.
 

The Anderson Time Reactor

Twisted spacetime around the earth, or
any rotating body, contains enormous
levels of potential energy. This is due
to the tension in the fabric of spacetime
caused by inertial frame-dragging.

Time-warped field theory shows how a properly configured energy beam can be used to initiate and maintain the coupling of two different areas of slightly twisted spacetime. This enables the discharge of significantly greater levels of stored potential energy and generates controllable fields of closed-timelike curves. The system that couples these two regions of different spacetime potential is common referred to as an Anderson Time Reactor or spacetime battery.

The Anderson Time Reactor is a system that couples two different areas of twisted spacetime, with two different spacetime tensions. The system can access and create a conduit to harvest that stored energy and through the coupling process create dense fields of Closed Timelike Curves (CTCs).

A reactor consists of a region of spacetime, large or small, surrounding a rotating mass, where inertial frame dragging effects are present twisting spacetime between two regions of space.

David Lewis Anderson

A specialized beam emitter, with a localized source nearer to the rotating mass, is directed toward a more distant region of space, across the region of twisted spacetime created by inertial frame-dragging.

A series of power collectors near and surrounding the beam emitter provide a conduit to then channel and control the received power. The resulting effect is that the potential energy in the twisted fabric of spacetime is coupled or bridged from the distant point to the local power collector array. The entire process is initiated and controlled by the system.

The Anderson Time Reactor system achieves this by using the application of Time-warped Field theory to create the ability to leak, tap into and control the greater energy stored in this spacetime tension (or energy potential difference), in between the distant point and the localized point in spacetime.

In the most basic terms, the Time Reactor can be looked at as a simple spacetime battery, accessing the significant potential energy that existing around any rotating body anywhere in spacetime.
 

Spacetime-Motive Force

Spectral image of energy pattern
near time reactor emitter and power
collector array showing coupling
and discharge of spacetime-motive
force including energy drift in the
direction of inertial frame dragging
of the Earth. New Mexico, USA, 2008

The coupling of these two points accesses what Dr. Anderson labeled a "spacetime-motive force" with the ability to produce high energy and time-warped fields allowing the containment and controlling of fields of closed-timelike curves.

The force between the localized and distant point is called the open spacetime-motive force. The open spacetime-motive force, even in the minimal effects of inertial frame-dragging, can be extremely large by present-day power generation standard standards. It is estimated that a single next-generation time reactor may have the ability to produce more than all of the worlds combined power generation capabilities today.

The amount of spacetime motive force depends on several factors.

These include the mass of the rotating body, its rotation speed, relative orientation of the two point to the axis of rotation, and the medium and distance between the localized and distant points in space. More simply, it is a function of the degree of inertial frame-dragging and the characteristics of the medium through which the Time Reactor must operate between the two regions to open a "discharge path."

Also, the amount of energy that is accessed, or time-warped fields generated, can be controlled in several ways through phasing and other characteristics of the emitter and power collector array.

A Practical Approach to Achieving Time Control

Practical time control and time travel requires significantly large energy levels, from some source, to operate effectively.

To achieve time control we can attempt to generate this large energy level or, as an alternative, access and channel the energy already existing and inherent in natural processes and the basic makeup or fabric of spacetime surrounding our planet.

As stated above, it is estimated that a single next-generation time reactor may have the ability to produce more than all of the world's combined power generation capabilities today.

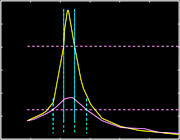
Time-warped field theory demonstrates
a practical way to generate the
necessary concentrated CTCs and
high power levels, without high input
power, for practical time control

The fabric of spacetime is elastic and very powerful. It takes a tremendous amount of power to create even the slightest twist in spacetime. One can think of the fabric of spacetime surrounding a rotating mass, like the Earth, to be a spring or a battery.

The rotating mass creates a twist in the fabric of spacetime who's natural state and desire is to unwind, just like a spring, or to discharge, just like a battery. Time-warped field technology uses relatively low input power to open a discharge path for this spacetime battery.

This technology itself does not create the energy levels required for time control and time travel. Instead, it relies on and operates using the energy stored within twisted spacetime around a rotating body that is created by the inertial frame-dragging effect. With only a small amount of system input power, time-warped field theory shows how enormous power levels can be accessed.

The coupling and discharge process, initiated and also defined by time-warped field theory and technology, generates significant levels of spacetime-motive force that can be used to generate very concentrated fields of closed-timelike curves near the Time Reactor's emitter and power collector array.

These fields of closed-timelike curves are concentrated and controllable and can permit both forward and backwards time control.

Back to Contents




Circulating Light Beams

Circulating Light Beams can be created using gamma and magnetic fields to warp time. The approach can twist space that causes time to be twisted, meaning you could theoretically walk through time as you walk through space.

A number of interesting post-Newtonian phenomena are known to occur for rotating distributions of matter in Einstein’s general theory of relativity.

Inertial frame dragging, for example, is a consequence of the weak gravitational field of a slowly rotating massive sphere. In addition, exact solutions of the Einstein field equations indicate the presence of closed timelike lines for rotating Kerr black holes, van Stockum rotating dust cylinders, and the rotating universe of Gödel.

The key characteristics of the application of circulating light beams for time control and time travel are presented in the picture below.

This is followed by more detail describing the approach below.

Recently, Ronald L. Mallett solved the linearized Einstein field equations to obtain the gravitational field produced by the electromagnetic radiation of a unidirectional ring laser.

It was shown that a massive spinning neutral particle at the center of the ring laser exhibited inertial frame dragging.

Ronald L Mallett

Traveling close to the speed of light will slow a clock, even an atomic clock. Likewise, a clock outside our atmosphere, far away from any gravitational pull, will run faster than a clock on earth. Therefore, if an artificial gravitational force were created, time travel would, in theory, be possible.

Mallett believes he has found a way to make it happen. By trapping light inside a photonic crystal, he can cause it to circulate. The energy of the circulating light will cause the space inside the circle to twist, causing a gravitational force.

This concept can be thought of as a spoon stirring a pot. The light is the spoon rotating around the inner rim of the pot. The space is the liquid being swirled by the spoon. As the space twists, it will coil the normally linear passage of time with it, spiraling the past, present, and future together into one continuous loop. It is this twisting of space and time that Mallett believes will make time travel possible.

Mallett and his partner at the University of Connecticut, Dr. Chandra Raychoudhuri, are seeking National Science Foundation funding for experiments that they hope will support their theories.

Their first experiment will be to trap light in a crystal and observe the reaction of a neutron inside the circle.

Mallett will insert polarized neutrons (neutrons that all spin in one direction) into the center of the circulating light.

If he sees a change in their spin he will know that space is indeed being twisted inside of the crystal. Should this experiment prove successful, the team will apply for funding to conduct studies to see if time bending is evident inside the circle of light.

Dr. Mark Silverman at Trinity College in nearby Hartford has suggested a possible way to see evidence of time bending: Two identical samples of a radioactive substance would be prepared with identical half-lives. One would be introduced into the time machine circulating in the same direction as the light, the other in the opposite direction.

If, at the end of the experiment, one sample had decayed further than the other, Mallett's theories of time travel would be supported.

Where the experiments will go from there is unclear.

There is a vast difference between slowing the decay rate of a radioactive particle and sending a human back in time. Science aside, sending people through time creates philosophical issues as well as physical ones. Consider the "Grandparent Paradox" in which a time traveler goes back in time and kills her grandparents, thus negating her entire existence. If she were never born, then she couldn't go back in time in the first place.

Mallett explains paradoxes such as these with a parallel-universe theory. He believes that with every decision we make, another version of us makes the opposite decision and splits off into a parallel universe. Thus the time traveler was born in the universe where she did not kill her grandparents.

This is where the line between philosophy and physics seems to blur.

"All of these things have their root in philosophy," says Mallett.

But he explains that the difference between physics and philosophy is experiment.

"All of these things would be philosophy without experimentation," he says.

True, the parallel-universe theory has not been directly supported by experiment, but Mallett uses the Heisenberg Uncertainty Principle to explain why the parallel universe theory is probable.

Heisenberg's Uncertainty Principle says that we cannot predict both the position of an electron and its spin at any given moment.

Without this principle,

"the universe should have collapsed immediately after it was formed," says Mallett.

A hydrogen atom, one of the building blocks of our universe, consists of a proton and an electron. Since the proton and electron have opposite charges they should be attracted to each other, collide, and destroy the atom.

But if that happened, we would know both the position of the electron (the point of impact with the proton) and its spin (none); therefore it is impossible for them to collide.

Sun distorting spacetime.

Similar to the Uncertainty Principle, quantum mechanics works on the theory that one can't make a definite prediction about anything that will happen next.

Therefore the parallel-universe theory works well. What will happen next can't be predicted because in fact, everything happens next.

It has long been known(3, 4) that the van Stockum solution for the exterior metric of an infinitely long rotating dust cylinder contains closed timelike lines. Dr. Mallett has proposed that closed timelike curves also occur for an infinitely long circulating cylinder of light. This model also shares some of the same limitations as the van Stockum solution in that the metric is not asymptotically flat, however, has emphasized that certain aspects of an infinitely long rotating dust cylinder may be shared by a long finite one.

This may also apply to a long but finite circulating cylinder of light.

Back to Contents




Wormholes

Since the 1930’s, physicists have speculated about the existence of "wormholes" in the fabric of space.

Wormholes are hypothetical areas of warped spacetime with great energy that can create tunnels through spacetime. if traversable would allow a traveler to quickly move through great distances in space and also travel through time. The difficulty lies in keeping the wormhole open while the traveler makes his journey: If the opening snaps shut, he will never survive to emerge at the other end.

For years, scientists believed that the transit was physically impossible.

But recent research, especially by the U.S. physicist Kip Thorne, suggests that it could be done using exotic materials capable of withstanding the immense forces involved. Even then, the time machine would be of limited use – for example, you could not return to a time before the wormhole was created.

Using wormhole technology would also require a society so technologically advanced that it could master and exploit the energy within black holes.

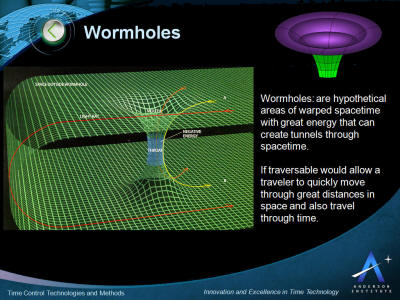
Hermann Weyl

Spacetime can be viewed as a 2D surface (to simplify understanding) that, when 'folded' over, allows the formation of a wormhole bridge.

A wormhole has at least two mouths that are connected to a single throat or tube. If the wormhole is traversable, then matter can 'travel' from one mouth to the other by passing through the throat.

While there is no observational evidence for wormholes, spacetime containing wormholes are known to be valid solutions in general relativity.

John Archibald Wheeler

The term wormhole was coined by the American theoretical physicist John Archibald Wheeler in 1957.

However, the idea of wormholes had already been theorized in 1921 by the German mathematician Hermann Weyl in connection with his analysis of mass in terms of electromagnetic field energy.

This analysis forces one to consider situations...where there is a net flux of lines of force through what topologists would call a handle of the multiply-connected space and what physicists might perhaps be excused for more vividly terming a ‘wormhole’.

The key characteristics of the application of wormholes for time control and time travel are presented in the picture below.

This is followed by more detail describing the science below.

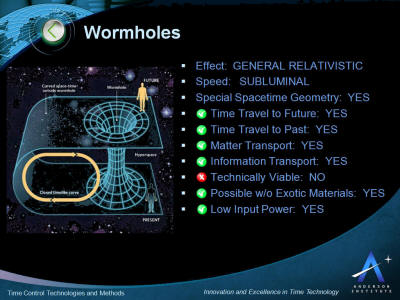
Definition

The basic notion of an intra-universe wormhole is that it is a compact region of spacetime whose boundary is topologically trivial but whose interior is not simply connected. Formalizing this idea leads to definitions such as the following, taken from Matt Visser's Lorentzian Wormholes.

If a Minkowski spacetime contains a compact region Ω, and if the topology of Ω is of the form Ω ~ R x Σ, where Σ is a three-manifold of nontrivial topology, whose boundary has topology of the form dΣ ~ S2, and if, furthermore, the hypersurfaces Σ are all spacelike, then the region Ω contains a quasi-permanent intra-universe wormhole.

Characterizing inter-universe wormholes is more difficult. For example, one can imagine a 'baby' universe connected to its 'parent' by a narrow 'umbilicus'. One might like to regard the umbilicus as the throat of a wormhole, but the spacetime is simply connected.

Schwarzschild wormholes

Diagram of a Schwarzschild Wormhole

Lorentzian wormholes known as Schwarzschild wormholes or Einstein-Rosen bridges are bridges between areas of space that can be modeled as vacuum solutions to the Einstein field equations by combining models of a black hole and a white hole.

This solution was discovered by Albert Einstein and his colleague Nathan Rosen, who first published the result in 1935. However, in 1962 John A. Wheeler and Robert W. Fuller published a paper showing that this type of wormhole is unstable, and that it will pinch off instantly as soon as it forms, preventing even light from making it through.

Before the stability problems of Schwarzschild wormholes were apparent, it was proposed that quasars were white holes forming the ends of wormholes of this type.

While Schwarzschild wormholes are not traversable, their existence inspired Kip Thorne to imagine traversable wormholes created by holding the 'throat' of a Schwarzschild wormhole open with exotic matter (material that has negative mass/energy).

Traversability

Wormholes would act as shortcuts
connecting distant regions of space-time.
By going through a wormhole, it might
be possible to travel between the two
regions faster than a beam of light
through normal space-time.

Lorentzian traversable wormholes would allow travel from one part of the universe to another part of that same universe very quickly or would allow travel from one universe to another.

The possibility of traversable wormholes in general relativity was first demonstrated by Kip Thorne and his graduate student Mike Morris in a 1988 paper; for this reason, the type of traversable wormhole they proposed, held open by a spherical shell of exotic matter, is referred to as a Morris-Thorne wormhole. Later, other types of traversable wormholes were discovered as allowable solutions to the equations of general relativity, including a variety analyzed in a 1989 paper by Matt Visser, in which a path through the wormhole can be made in which the traversing path does not pass through a region of exotic matter.

However in the pure Gauss-Bonnet theory exotic matter is not needed in order for wormholes to exist- they can exist even with no matter. A type held open by negative mass cosmic strings was put forth by Visser in collaboration with Cramer et al., in which it was proposed that such wormholes could have been naturally created in the early universe.

Wormholes connect two points in spacetime, which means that they would in principle allow travel in time, as well as in space. In 1988, Morris, Thorne and Yurtsever worked out explicitly how to convert a wormhole traversing space into one traversing time.[4]

However, it has been said a time traversing wormhole cannot take you back to before it was made but this is disputed.

Faster-than-light travel

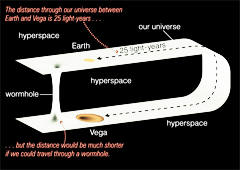
Special relativity only applies locally.

Wormholes allow superluminal (faster-than-light) travel by ensuring that the speed of light is not exceeded locally at any time. While traveling through a wormhole, subluminal (slower-than-light) speeds are used. If two points are connected by a wormhole, the time taken to traverse it would be less than the time it would take a light beam to make the journey if it took a path through the space outside the wormhole.

However, a light beam traveling through the wormhole would always beat the traveler. As an analogy, running around to the opposite side of a mountain at maximum speed may take longer than walking through a tunnel crossing it.

You can walk slowly while reaching your destination more quickly because the distance is smaller.

Time travel

A wormhole could allow time travel.

This could be accomplished by accelerating one end of the wormhole to a high velocity relative to the other, and then sometime later bringing it back; relativistic time dilation would result in the accelerated wormhole mouth aging less than the stationary one as seen by an external observer, similar to what is seen in the twin paradox.

However, time connects differently through the wormhole than outside it, so that synchronized clocks at each mouth will remain synchronized to someone traveling through the wormhole itself, no matter how the mouths move around. This means that anything which entered the accelerated wormhole mouth would exit the stationary one at a point in time prior to its entry.

For example, consider two clocks at both mouths both showing the date as 2000.

After being taken on a trip at relativistic velocities, the accelerated mouth is brought back to the same region as the stationary mouth with the accelerated mouth's clock reading 2005 while the stationary mouth's clock read 2010. A traveler who entered the accelerated mouth at this moment would exit the stationary mouth when its clock also read 2005, in the same region but now five years in the past.

Such a configuration of wormholes would allow for a particle's world line to form a closed loop in spacetime, known as a closed timelike curve.

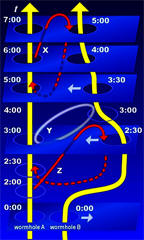
It is thought that it may not be possible to convert a wormhole into a time machine in this manner; some analyses using the semi-classical approach to incorporating quantum effects into general relativity indicate that a feedback loop of virtual particles would circulate through the wormhole with ever-increasing intensity, destroying it before any information could be passed through it, in keeping with the chronology protection conjecture.

This has been called into question by the suggestion that radiation would disperse after traveling through the wormhole, therefore preventing infinite accumulation. The debate on this matter is described by Kip S. Thorne in the book Black Holes and Time Warps. There is also the Roman ring, which is a configuration of more than one wormhole.

This ring seems to allow a closed time loop with stable wormholes when analyzed using semi-classical gravity, although without a full theory of quantum gravity it is uncertain whether the semi-classical approach is reliable in this case.

Metrics

Theories of wormhole metrics describe the spacetime geometry of a wormhole and serve as theoretical models for time travel. An example of a (traversable) wormhole metric is the following:

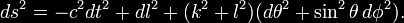
One type of non-traversable wormhole metric is the Schwarzschild solution:

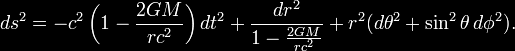
In fiction

Wing Commander ships are configured
with jump drives to propel a spacecraft
between two connecting stellar systems.

Wormholes are features of science fiction as they allow interstellar (and sometimes inter-universal) travel within human timescales.

It is common for the creators of a fictional universe to decide that faster-than-light travel is either impossible or that the technology does not yet exist, but to use wormholes as a means of allowing humans to travel long distances in short periods. Military science fiction (such as the Wing Commander games) often uses a "jump drive" to propel a spacecraft between two fixed "jump points" connecting stellar systems.

Connecting systems in a network like this results in a fixed "terrain" with choke points that can be useful for constructing plots related to military campaigns. The Alderson points used by Larry Niven and Jerry Pournelle in The Mote in God's Eye and related novels are an example, although the mechanism does not seem to describe actual wormhole physics.

David Weber has also used the device in the Honorverse and other books such as those based upon the Starfire universe. Naturally occurring wormholes form the basis for interstellar travel in Lois McMaster Bujold's Vorkosigan Saga. They are also used to create an Interstellar Commonwealth in Peter F. Hamilton's Commonwealth Saga.

In Jack L. Chalker's The Rings of the Master series, interstellar class spaceships are capable of calculating complex equations and punching Wormholes in the fabric of the Universe in order to enable rapid travel.

Concept of wormholes is used in The Wild Blue Yonder, a science fiction film by Werner Herzog.

Mass Relay Map in the Video Game Mars Effect

The Mass Relays in the videogame Mass Effect can be perceived as stabilized wormholes that allow for near instantaneous, "faster-than-light" travel from one end to the other.

The Massively Multiplayer Online Game EVE Online utilizes wormholes extensively as they are created in the use of the stargate technology which allows for interstellar travel in the game world.

The Vega Strike first-person space trading and combat simulator features wormholes to travel through star systems. The engine is open-source and has various mods and total conversions which have wormholes too, like Vega Trek, a Vega Strike mod based on the Star Trek universe.

Or the Privateer Remake, a remake of Wing Commander: Privateer.

Bajoran Wormhole in Star Trek

Wormholes also play pivotal roles in science fiction where faster-than-light travel is possible though limited, allowing connections between regions that would be otherwise unreachable within conventional timelines.

Several examples appear in the Star Trek franchise, including the Bajoran wormhole in the Deep Space Nine series. In 1979's Star Trek: The Motion Picture the USS Enterprise was trapped in an artificial wormhole caused by an imbalance in the calibration of the ship's warp engines when it first achieved faster-than-light speed.

In the Star Trek: Voyager series, the cybernetic species the Borg use what, in the Star Trek universe, are referred to as transwarp conduits, allowing ships to move nearly instantaneously to any part of the galaxy in which an exit aperture exists. Although these conduits are never described as "wormholes", they appear to share several traits in common with them.

The 1979 Disney film The Black Hole's plot centers around a massive black hole, although it makes virtually no use of then-current worm-hole physics, with only one rather desultory mention of an Einstein-Rosen bridge.

A trip through the black hole turns theological, abandoning scientific rationale.

Wormhole Transporter in the
movie Contact.

In Carl Sagan's novel Contact and subsequent 1997 film starring Jodie Foster and Matthew McConaughey, Foster's character Ellie travels 26 light years through a series of wormholes to the star Vega.

The round trip, which to Ellie lasts 18 hours, passes by in a fraction of a second on Earth, making it appear she went nowhere. In her defense, Foster mentions an Einstein-Rosen bridge and tells how she was able to travel faster than light and time. Analysis of the situation by Kip Thorne, on the request of Sagan, is quoted by Thorne as being his original impetus for analyzing the physics of wormholes.

Wormholes play major roles in the television series Farscape, where they are the cause of John Crichton's presence in the far reaches of our own galaxy, and in the Stargate series, where stargates create a stable artificial wormhole where matter is dematerialized, converted into energy, and is sent through to be rematerialized at the other side.

In the latter series, the devices were discovered in Egypt by an archeologist, and were built by aliens known as the Ancients or the Alterans. In the science fiction series Sliders, a wormhole (or vortex, as it is usually called in the show) is used to travel between parallel worlds, and one is seen at least once or twice in every episode.

In the pilot episode it was referred to as an "Einstein-Rosen-Podolsky bridge".

Wormhole in movie Donnie Darko

The central theme in the movie Donnie Darko revolves around Einstein-Rosen bridges.

It is possible that the Webway technology used by the Eldar of the fictional Warhammer 40,000 could be perceived as wormhole technology.

In Command & Conquer 3 and in its expansion the Scrin faction (an alien life form with unknown origins from outer solar system) uses artificial wormholes for military purposes to convey infantry and vehicles behind enemy lines.

In the Invader Zim episode, "A Room with a Moose" Zim utilizes a wormhole to send his classmates into a parallel universe that consists entirely of a room with a large moose inside it.

The television series Strange Days at Blake Holsey High is about a wormhole the science club found at their school.

In an episode called "wormhole" in the 13th season of the long running American series Power Rangers, called Power Rangers SPD the spd rangers go through a wormhole to team up with the previous team of Power Rangers Dino Thunder from year 2004, after their enemy Emperor Grumm goes through one.

In the video game "Supreme Commander" the UEF faction utilizes aether-gates for long distance military strikes.

Black hole in video game Spore

In the video game "Spore", the player can travel through various black holes, which act as wormholes for the player to go to its counterpart located usually on the other side of the galaxy; something that would take much longer to do by flying there manually.

In the 1995-1996 FOX military science fiction series SPACE: Above and Beyond, during the first several episodes, the United Earth Force travel through wormholes, called the "Kali Region" or "Galileo Region" to arrive at exo-solar destinations. This idea is abandoned after the second episode.

In the movie Race to Witch Mountain the 2 aliens from a planet which is 3000 light years away from Earth use wormholes to travel to Earth.

In the 2009 Doctor Who Easter special, Planet of the Dead, the Doctor and a group of passengers aboard a double-decker bus are transported to an alien world via a wormhole.

Back to Contents

 

Cosmic Strings

Cosmic Strings are a hypothetical 1-dimensional (spatially) topological defect in the fabric of spacetime left over from the formation of the universe. Interaction could create fields of closed time-like curves permitting backwards time travel.

Some scientists have suggested using "cosmic strings" to construct a time machine. By maneuvering two cosmic strings close together – or possibly just one string plus a black hole – it is theoretically possible to create a whole array of "closed time-like curves." Your best bet is to fire two infinitely long cosmic strings past each other at very high speeds, then fly your ship around them in a carefully calculated figure eight. In theory, you would be able to emerge anywhere, anytime!

At the moment, these are purely theoretical objects that might possibly be left over from the creation of the universe in the Big Bang. A black hole contains a one-dimensional singularity – an infinitely small point in the space-time continuum. A cosmic string, if such a thing existed, would be a two-dimensional infinitely thin line that has even stranger effects on the fabric of space and time.

Although no one has actually found a cosmic string, astronomers have suggested that they may explain strange effects seen in distant galaxies.

A cosmic string is a 1-dimensional (spatially) topological defect in various fields.

Cosmic strings are hypothesized to form when the field undergoes a phase change in different regions of spacetime, resulting in condensations of energy density at the boundaries between regions. This is somewhat analogous to the imperfections that form between crystal grains in solidifying liquids, or the cracks that form when water freezes into ice.

The phase changes that produce cosmic strings may have occurred in the earliest moments of the universe's evolution.

The key characteristics of the application of cosmic strings for time control and time travel are presented in the picture below. This is followed by more detail describing the theory below.

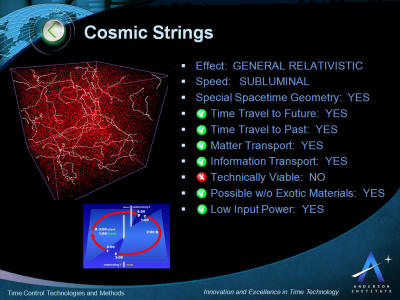
Cosmic strings, if they exist, would be extremely thin with diameters on the same order as a proton.

They would have immense density, however, and so would represent significant gravitational sources. A cosmic string 1.6 kilometers in length may be heavier than the Earth. However general relativity predicts that the gravitational potential of a straight string vanishes: there is no gravitational force on static surrounding matter.

The only gravitational effect of a straight cosmic string is a relative deflection of matter (or light) passing the string on opposite sides (a purely topological effect). A closed loop of cosmic string gravitates in a more conventional way. During the expansion of the universe, cosmic strings would form a network of loops, and their gravity could have been responsible for the original clumping of matter into galactic superclusters.

A cosmic string's vibrations, which would oscillate near the speed of light, can cause part of the string to pinch off into an isolated loop. These loops have a finite lifespan due to decay via gravitational radiation.

Other types of topological defects in spacetime are domain walls, monopoles, and textures.

Observational evidence

It was once thought that the gravitational influence of cosmic strings might contribute to the large-scale clumping of matter in the universe, but all that is known today through galaxy surveys and precision measurements of the cosmic microwave background fits an evolution out of random, Gaussian fluctuations.

These precise observations therefore tend to rule out a significant role for cosmic strings.

Gravitational lensing of a galaxy by a straight section of a cosmic string would produce two identical, undistorted images of the galaxy.

In 2003 a group led by Mikhail Sazhin reported the accidental discovery of two seemingly identical galaxies very close together in the sky, leading to speculation that a cosmic string had been found. However, observations by the Hubble Space Telescope in January 2005 showed them to be a pair of similar galaxies, not two images of the same galaxy.

A cosmic string would produce a similar duplicate image of fluctuations in the cosmic microwave background, which might be detectable by the upcoming Planck Surveyor mission.

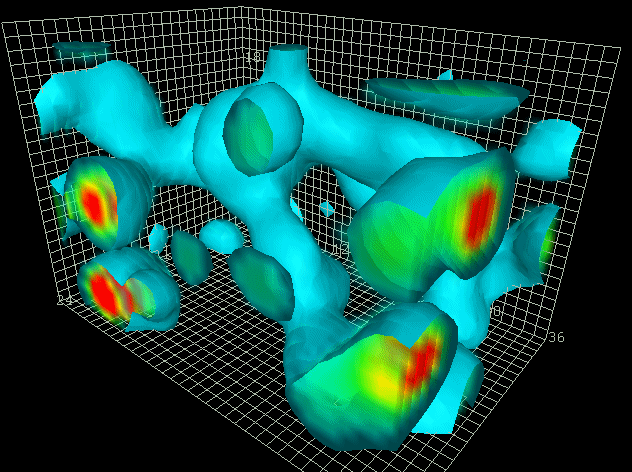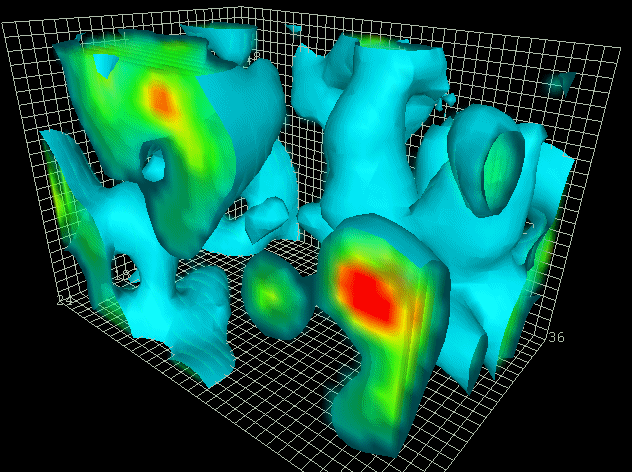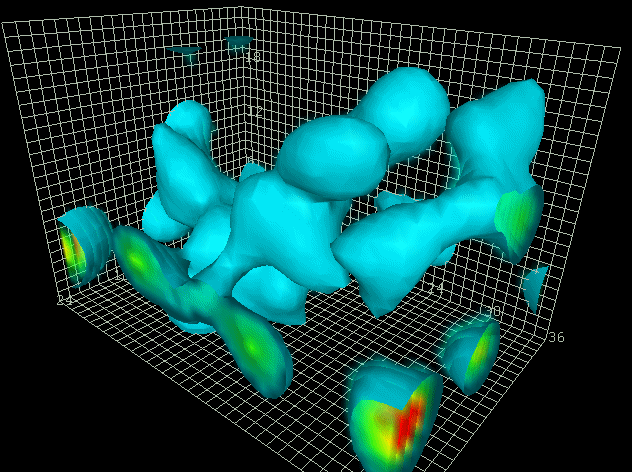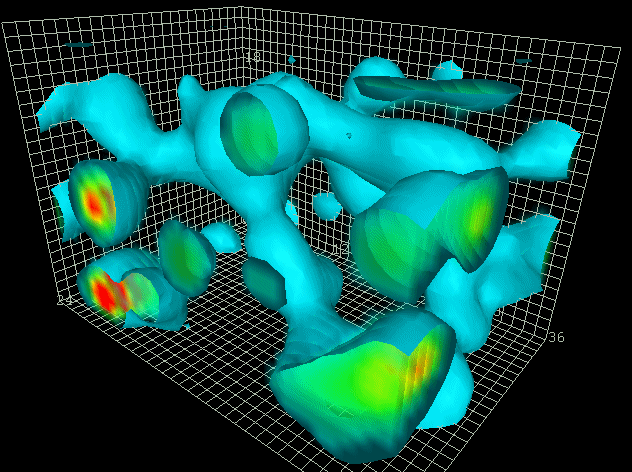
A second piece of evidence supporting cosmic string theory is a phenomenon observed in observations of the "double quasar" called Q0957+561A,B.

Originally discovered by Dennis Walsh, Bob Carswell, and Ray Weymann in 1979, the double image of this quasar is caused by a galaxy positioned between it and the Earth. The gravitational lens effect of this intermediate galaxy bends the quasar's light so that it follows two paths of different lengths to Earth. The result is that we see two images of the same quasar, one arriving a short time after the other (about 417.1 days later).

However, a team of astronomers at the Harvard-Smithsonian Center for Astrophysics led by Rudolph Schild studied the quasar and found that during the period between September 1994 and July 1995 the two images appeared to have no time delay; changes in the brightness of the two images occurred simultaneously on four separate occasions.

Schild and his team believe that the only explanation for this observation is that a cosmic string passed between the Earth and the quasar during that time period traveling at very high speed and oscillating with a period of about 100 days.

The Laser Interferometer Gravitational-Wave Observatory (LIGO) and upcoming gravitational wave observatories will search for cosmic strings as well as other phenomenon with the byproduct of gravitational waves.

String theory and cosmic strings

There is no direct connection between string theory and the theory of cosmic strings (the names were chosen independently by analogy with ordinary string).

However, work in string theory revived interest in cosmic strings in the early 2000s. In 2002 Henry Tye and collaborators observed the production of cosmic strings during the last stages of brane inflation. It was also pointed out by string theorist Joseph Polchinski that the expanding Universe could have stretched a "fundamental" string (the sort which superstring theory considers) until it was of intergalactic size.

Such a stretched string would exhibit many of the properties of the old "cosmic" string variety, making the older calculations useful again. Furthermore, modern superstring theories offer other objects which could feasibly resemble cosmic strings, such as highly elongated one-dimensional D-branes (known as "D-strings").

As theorist Tom Kibble remarks,

"string theory cosmologists have discovered cosmic strings lurking everywhere in the undergrowth".

Older proposals for detecting cosmic strings could now be used to investigate superstring theory.

Scientists at the LIGO Livingston Observatory in
Louisiana are searching for evidence of
gravitational waves.

Superstrings, D-strings or other stringy objects stretched to intergalactic scales would radiate gravitational waves, which could presumably be detected using experiments like LIGO. They might also cause slight irregularities in the cosmic microwave background, too subtle to have been detected yet but possibly within the realm of future observability.

Note that most of these proposals depend, however, on the appropriate cosmological fundamentals (strings, branes, etc.), and no convincing experimental verification of these has been performed.

Back to Contents

 

Tipler Cylinder

A Tipler Cylinder uses a massive and long cylinder spinning around its longitudinal axis. The rotation creates a frame-dragging effect and fields of closed time-like curves traversable in a way to achieve subluminal time travel to the past.

Civilizations with the technology to harness black holes might be better advised to leave wormholes alone and try the time-warp method suggested by U.S. astronomer Frank Tipler. He has a simple recipe for a time machine: First take a piece of material 10 time the mass of the Sun, squeeze it together and roll it into a long, thin, super-dense cylinder – a bit like a black hole that has passed through a spaghetti factory. Then spin the cylinder up to a few billion revolutions per minute and see what happens.

Tipler predicts that a ship following a carefully plotted spiral course around the cylinder would immediately find itself on a "closed, time-like curve."

It would emerge thousands, even billions, of years from its starting point and possibly several galaxies away. There are problems, though. For the mathematics to work properly, Tipler’s cylinder has to be infinitely long. Also, odd things happen near the ends and you need to steer well clear of them in your timeship.

However, if you make the device as long as you can, and stick to paths close to the middle of the cylinder, you should survive the trip!

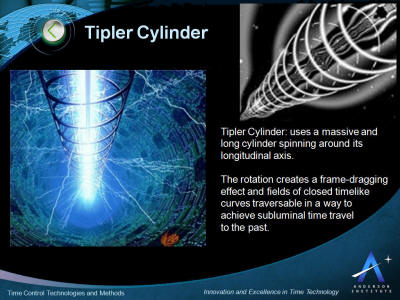
The Tipler cylinder, also called a Tipler time machine, is a hypothetical object theorized to be a potential mode of time travel - an approach that is conceivably functional within humanity's current understanding of physics, specifically the theory of general relativity, although later results have shown that a Tipler cylinder could only allow time travel if its length would appear infinite.

The key characteristics of the application of Tipler Cylinders for time control and time travel are presented in the picture below. This is followed by more detail describing the approach below.

Frank Tipler

The Tipler cylinder was discovered as a solution to the equations of general relativity by Willem Jacob van Stockum in 1936 and Kornel Lanczos in 1924, but not recognized as allowing closed timelike curves until an analysis by Frank Tipler in 1974.

Tipler showed in his 1974 paper, "Rotating Cylinders and the Possibility of Global Causality Violation" that in a spacetime containing a massive, infinitely long cylinder which was spinning along its longitudinal axis, the cylinder should create a frame-dragging effect.

This frame-dragging effect warps spacetime in such a way that the light cones of objects in the cylinder's proximity become tilted, so that part of the light cone then points backwards along the time axis on a space time diagram.

Therefore a spacecraft accelerating sufficiently in the appropriate direction can travel backwards through time along a closed timelike curve or CTC.

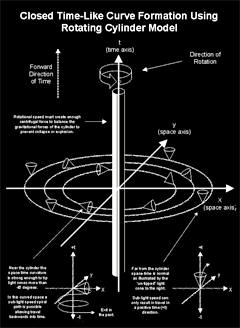
Closed timelike curve formation
using rotating cylinder model

CTC's are associated, in Lorentzian manifolds which are interpreted physically as spacetimes, with the possibility of causal anomalies such as going back in time and potentially shooting your own grandfather, although paradoxes might be avoided using some constraint such as the Novikov self-consistency principle.

They have an unnerving habit of appearing in some of the most important exact solutions in general relativity, including the Kerr vacuum (which models a rotating black hole) and the van Stockum dust (which models a cylindrically symmetrical configuration of rotating pressureless fluid or dust).

An objection to the practicality of building a Tipler cylinder was discovered by Stephen Hawking, who posited a conjecture showing that according to general relativity it is impossible to build a time machine in any finite region that satisfies the weak energy condition, meaning that the region contains no exotic matter with negative energy.

The Tipler cylinder, on the other hand, does not involve any negative energy.

Tipler's original solution involved a cylinder of infinite length, which is easier to analyze mathematically, and although Tipler suggested that a finite cylinder might produce closed timelike curves if the rotation rate were fast enough, he did not prove this.

A spirallohedron of 6 hyperstrings
from 6 parallel universes

But Hawking argues that because of his conjecture,

"it can't be done with positive energy density everywhere! I can prove that to build a finite time machine, you need negative energy."

Hawking's proof appears in his 1992 paper on the chronology protection conjecture, where he examines,

"the case that the causality violations appear in a finite region of spacetime without curvature singularities" and proves that "there will be a Cauchy horizon that is compactly generated and that in general contains one or more closed null geodesics which will be incomplete. One can define geometrical quantities that measure the Lorentz boost and area increase on going round these closed null geodesics. If the causality violation developed from a noncompact initial surface, the averaged weak energy condition must be violated on the Cauchy horizon."

Back to Contents

 

Casimir Effect

The Casimer Effect is a physical force arising from a quantized field, for example between two uncharged plates. This can produce a locally mass-negative region of space-time that could stabilize a wormhole to allow faster than light travel.

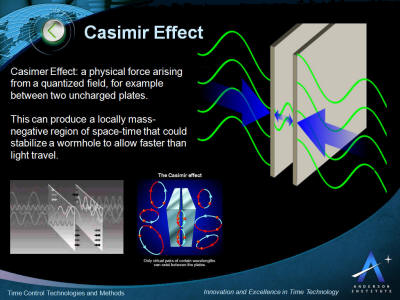
In quantum field theory, the Casimir effect and the Casimir-Polder force are physical forces arising from a quantized field.

The typical example is of two uncharged metallic plates in a vacuum, placed a few micrometers apart, without any external electromagnetic field. In a classical description, the lack of an external field also means that there is no field between the plates, and no force would be measured between them.

When this field is instead studied using quantum electrodynamics, it is seen that the plates do affect the virtual photons which constitute the field, and generate a net force - either an attraction or a repulsion depending on the specific arrangement of the two plates.

The key characteristics of the application of the Casimir Effect for time control and time travel are presented in the picture below.

This is followed by more detail describing the effect below.

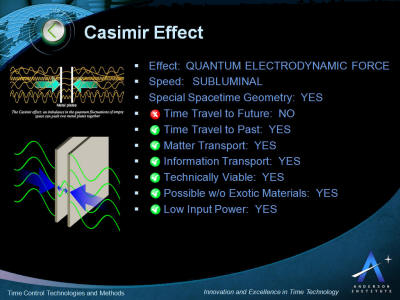
Although the Casimir effect can be expressed in terms of virtual particles interacting with the objects, it is best described and more easily calculated in terms of the zero-point energy of a quantized field in the intervening space between the objects.

This force has been measured, and is a striking example of an effect purely due to second quantization. However, the treatment of boundary conditions in these calculations has led to some controversy. In fact "Casimir's original goal was to compute the van der Waals force between polarizable molecules" of the metallic plates.

Thus it can be interpreted without any reference to the zero-point energy (vacuum energy) or virtual particles of quantum fields.

Hendrik Casimir

Dutch physicists Hendrik B. G. Casimir and Dirk Polder proposed the existence of the force and formulated an experiment to detect it in 1948 while participating in research at Philips Research Labs.

The classic form of the experiment, described above, successfully demonstrated the force to within 15% of the value predicted by the theory.

Because the strength of the force falls off rapidly with distance, it is only measurable when the distance between the objects is extremely small.

On a submicrometre scale, this force becomes so strong that it becomes the dominant force between uncharged conductors. In fact, at separations of 10 nm - about 100 times the typical size of an atom - the Casimir effect produces the equivalent of 1 atmosphere of pressure (101.3 kPa), the precise value depending on surface geometry and other factors.

In modern theoretical physics, the Casimir effect plays an important role in the chiral bag model of the nucleon; and in applied physics, it is significant in some aspects of emerging microtechnologies and nanotechnologies.

Vacuum energy

The causes of the Casimir effect are described by quantum field theory, which states that all of the various fundamental fields, such as the electromagnetic field, must be quantized at each and every point in space.

In a simplified view, a "field" in physics may be envisioned as if space were filled with interconnected vibrating balls and springs, and the strength of the field can be visualized as the displacement of a ball from its rest position.

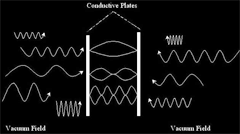
Vibrations in this field propagate and are governed by the appropriate wave equation for the particular field in question.

The second quantization of quantum field theory requires that each such ball-spring combination be quantized, that is, that the strength of the field be quantized at each point in space. Canonically, the field at each point in space is a simple harmonic oscillator, and its quantization places a quantum harmonic oscillator at each point. Excitations of the field correspond to the elementary particles of particle physics. However, even the vacuum has a vastly complex structure, all calculations of quantum field theory must be made in relation to this model of the vacuum.

The vacuum has, implicitly, all of the properties that a particle may have: spin, or polarization in the case of light, energy, and so on. On average, all of these properties cancel out: the vacuum is, after all, "empty" in this sense. One important exception is the vacuum energy or the vacuum expectation value of the energy.

The quantization of a simple harmonic oscillator states that the lowest possible energy or zero-point energy that such an oscillator may have is

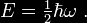
Summing over all possible oscillators at all points in space gives an infinite quantity.

To remove this infinity, one may argue that only differences in energy are physically measurable; this argument is the underpinning of the theory of renormalization. In all practical calculations, this is how the infinity is always handled. In a deeper sense, however, renormalization is unsatisfying, and the removal of this infinity presents a challenge in the search for a Theory of Everything.

Currently there is no compelling explanation for how this infinity should be treated as essentially zero; a non-zero value is essentially the cosmological constant and any large value causes trouble in cosmology.

The Casimir Effect

Simulation of Casimir Force

Casimir's observation was that the second-quantized quantum electromagnetic field, in the presence of bulk bodies such as metals or dielectrics, must obey the same boundary conditions that the classical electromagnetic field must obey. In particular, this affects the calculation of the vacuum energy in the presence of a conductor or dielectric.

Consider, for example, the calculation of the vacuum expectation value of the electromagnetic field inside a metal cavity, such as, for example, a radar cavity or a microwave waveguide.

In this case, the correct way to find the zero point energy of the field is to sum the energies of the standing waves of the cavity. To each and every possible standing wave corresponds an energy; say the energy of the nth standing wave is En. The vacuum expectation value of the energy of the electromagnetic field in the cavity is then

with the sum running over all possible values of n enumerating the standing waves. The factor of 1/2 corresponds to the fact that the zero-point energies are being summed - it is the same 1/2 as appears in the equation...

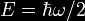
Written in this way, this sum is clearly divergent; however, it can be used to create finite expressions.

In particular, one may ask how the zero point energy depends on the shape s of the cavity. Each energy level En depends on the shape, and so one should write En(s) for the energy level, and,

for the vacuum expectation value. At this point comes an important observation: the force at point p on the wall of the cavity is equal to the change in the vacuum energy if the shape s of the wall is perturbed a little bit, say by δs, at point p.

That is, one has

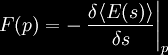
This value is finite in many practical calculations.

Casimir's calculation

In the original calculation done by Casimir, he considered the space between a pair of conducting metal plates at distance a apart.

In this case, the standing waves are particularly easy to calculate, since the transverse component of the electric field and the normal component of the magnetic field must vanish on the surface of a conductor. Assuming the parallel plates lie in the x-y plane, the standing waves are

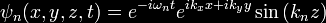
where ψ stands for the electric component of the electromagnetic field, and, for brevity, the polarization and the magnetic components are ignored here. Here,
kx and ky are the wave vectors in directions parallel to the plates, and

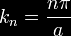
is the wave-vector perpendicular to the plates. Here, n is an integer, resulting from the requirement that ψ vanish on the metal plates. The energy of this wave is

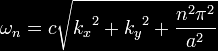
where c is the speed of light. The vacuum energy is then the sum over all possible excitation modes

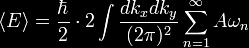
where A is the area of the metal plates, and a factor of 2 is introduced for the two possible polarizations of the wave. This expression is clearly infinite, and to proceed with the calculation, it is convenient to introduce a regulator (discussed in greater detail below). The regulator will serve to make the expression finite, and in the end will be removed. The zeta-regulated version of the energy per unit-area of the plate is

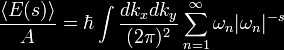
In the end, the limit

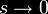
is to be taken. Here s is just a complex number, not to be confused with the shape discussed previously. This integral/sum is finite for s real and larger than 3. The sum has a pole at s=3, but may be analytically continued to s=0, where the expression is finite. Expanding this, one gets

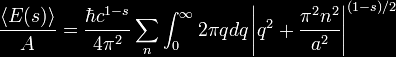
where polar coordinates

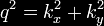
were introduced to turn the double integral into a single integral. The q in front is the Jacobian, and the 2π comes from the angular integration. The integral is easily performed, resulting in

The sum may be understood to be the Riemann zeta function, and so one has

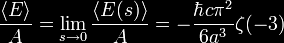
But ζ( − 3) = 1 / 120 and so one obtains

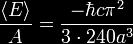
The Casimir force per unit area,

Fc / A for idealized, perfectly conducting plates with vacuum between them is

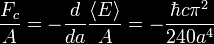
where

(hbar, ħ) is the reduced Planck constant,
c is the speed of light,
a is the distance between the two plates.

The force is negative, indicating that the force is attractive: by moving the two plates closer together, the energy is lowered. The presence of shows that the Casimir force per unit area Fc / A is very small, and that furthermore, the force is inherently of quantum-mechanical origin.

More recent theory

 

 

Concept of zero-point energy module
using the Casimir Effect

 

A very complete analysis of the Casimir effect at short distances is based upon a detailed analysis of the van der Waals force by Lifshitz. Using this approach, complications of the bounding surfaces, such as the modifications to the Casimir force due to finite conductivity, can be calculated numerically using the tabulated complex dielectric functions of the bounding materials.

 

In addition to these factors, complications arise due to surface roughness of the boundary and to geometry effects such as degree of parallelism of bounding plates. For boundaries at large separations, retardation effects give rise to a long-range interaction. For the case of two parallel plates composed of ideal metals in vacuum, the results reduce to Casimir’s.
Measurement

One of the first experimental tests was conducted by Marcus Sparnaay at Philips in Eindhoven, in 1958, in a delicate and difficult experiment with parallel plates, obtaining results not in contradiction with the Casimir theory, but with large experimental errors.

The Casimir effect was measured more accurately in 1997 by Steve K. Lamoreaux of Los Alamos National Laboratory and by Umar Mohideen and Anushree Roy of the University of California at Riverside.

 

In practice, rather than using two parallel plates, which would require phenomenally accurate alignment to ensure they were parallel, the experiments use one plate that is flat and another plate that is a part of a sphere with a large radius. In 2001, a group at the University of Padua finally succeeded in measuring the Casimir force between parallel plates using microresonators.

 

Regularisation
In order to be able to perform calculations in the general case, it is convenient to introduce a regulator in the summations. This is an artificial device, used to make the sums finite so that they can be more easily manipulated, followed by the taking of a limit so as to remove the regulator.

The heat kernel or exponentially regulated sum is

 

 

 

 

where the limit

 

 

 

 

is taken in the end. The divergence of the sum is typically manifested as

 

 

 

 

for three-dimensional cavities. The infinite part of the sum is associated with the bulk constant C which does not depend on the shape of the cavity. The interesting part of the sum is the finite part, which is shape-dependent. The Gaussian regulator

 

 

 

 

is better suited to numerical calculations because of its superior convergence properties, but is more difficult to use in theoretical calculations. Other, suitably smooth, regulators may be used as well. The zeta function regulator

 

 

 

 

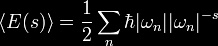
is completely unsuited for numerical calculations, but is quite useful in theoretical calculations. In particular, divergences show up as poles in the complex s plane, with the bulk divergence at s=4.

 

This sum may be analytically continued past this pole, to obtain a finite part at s=0.

Not every cavity configuration necessarily leads to a finite part (the lack of a pole at s=0) or shape-independent infinite parts. In this case, it should be understood that additional physics has to be taken into account. In particular, at extremely large frequencies (above the plasma frequency), metals become transparent to photons (such as x-rays), and dielectrics show a frequency-dependent cutoff as well.

 

This frequency dependence acts as a natural regulator. There are a variety of bulk effects in solid state physics, mathematically very similar to the Casimir effect, where the cutoff frequency comes into explicit play to keep expressions finite. (These are discussed in greater detail in Landau and Lifshitz, "Theory of Continuous Media".)

 

Generalities

 

 

experimental setup for the conversion of
vacuum energy into mechanical-energy.

 

The Casimir effect can also be computed using the mathematical mechanisms of functional integrals of quantum field theory, although such calculations are considerably more abstract, and thus difficult to comprehend. In addition, they can be carried out only for the simplest of geometries.

 

However, the formalism of quantum field theory makes it clear that the vacuum expectation value summations are in a certain sense summations over so-called "virtual particles".

 

More interesting is the understanding that the sums over the energies of standing waves should be formally understood as sums over the eigenvalues of a Hamiltonian. This allows atomic and molecular effects, such as the van der Waals force, to be understood as a variation on the theme of the Casimir effect.

 

Thus one considers the Hamiltonian of a system as a function of the arrangement of objects, such as atoms, in configuration space. The change in the zero-point energy as a function of changes of the configuration can be understood to result in forces acting between the objects.

 

In the chiral bag model of the nucleon, the Casimir energy plays an important role in showing the mass of the nucleon is independent of the bag radius. In addition, the spectral asymmetry is interpreted as a non-zero vacuum expectation value of the baryon number, cancelling the topological winding number of the pion field surrounding the nucleon.

 

Casimir effect and wormholes
Exotic matter with negative energy density is required to stabilize a wormhole. Morris, Thorne and Yurtsever pointed out that the quantum mechanics of the Casimir effect can be used to produce a locally mass-negative region of space-time, and suggested that negative effect could be used to stabilize a wormhole to allow faster than light travel. This concept has been used extensively in Science Fiction.

 

Analogies
A similar analysis can be used to explain Hawking radiation that causes the slow "evaporation" of black holes (although this is generally visualized as the escape of one particle from a virtual particle-antiparticle pair, the other particle having been captured by the black hole).

 

Repulsive forces
There are few instances wherein the Casimir effect can give rise to repulsive forces between uncharged objects. In a seminal paper, Evgeny Lifshitz showed (theoretically) that in certain circumstances (most commonly involving liquids), repulsive forces can arise. This has sparked interest in applications of the Casimir effect toward the development of levitating devices.

 

Other scientists have also suggested the use of gain media to achieve a similar levitation effect, though this is controversial because these materials seem to violate fundamental causality constraints and the requirement of thermodynamic equilibrium. An experimental demonstration of the Casimir-based levitation was recently demonstrated by the Capasso group at Harvard through experiments involving a gold-coated particle and silica thin film immersed in bromobenzene.

 

Applications
It has been suggested that the Casimir forces have application in nanotechnology, in particular silicon integrated circuit technology based micro- and nanoelectromechanical systems, and so-called Casimir oscillators.

 

Classical 'Critical' Casimir Effect
In 2008, physicists in Germany made the first direct measurements of the “critical Casimir effect”, a classical analogue of the quantum Casimir effect. This effect had been theoretically predicted in 1978 by Michael Fisher and Pierre-Gilles de Gennes but all observations had been indirect.

In this experiment, the critical Casimir effect arises in a mixed liquid that is close to its critical point. The liquid used was a solution of water and the oil 2,6-lutidine which has a critical point of 34°C at normal atmospheric pressure. As this liquid approaches its critical point, the oil and water starts separate into small regions whose size and shape are subject to statistical fluctuations and that exhibit random Brownian motion.

 

To demonstrate the effect, a tiny coated Styrofoam ball is suspended in the liquid close to the wall of its coated glass container. The ball and the container coatings are the same and both have a preference for either oil or water. As the liquid nears its critical point, total internal reflection microscopy is used to detect displacements of the ball.

 

From the sudden movements detected only towards the glass, the classical Casimir force was calculated to be approximately 600 fN (6 x 10−13 N). To tune the effect for repulsion, the coatings of the glass and the ball are changed so that one prefers oil and the other water.

While the German physicists say this reverse critical Casimir effect could be useful in nanoelectromechanical systems, its dependence upon a very specific temperature presently limits its usefulness.

Back to Contents