|
from 21stCenturyScienceTech Website
By the end of this piece you shall have been given the essential concepts and facts both to understand this ugly truth, and to act to prevent it.
Gustave Doré
illustration of Don Quixote, 1863
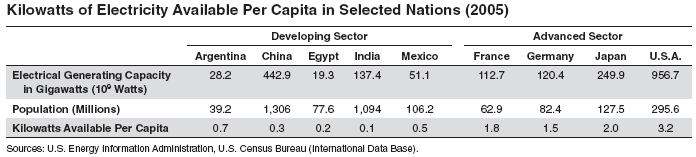
Begin with this: To maintain a global population in a condition resembling a modern 21st-Century standard of living will require an installed electrical generating capacity of at least 3 to 5 kilowatts per capita.
Today, only the United States, Japan,
and a few countries of western Europe even approximate this level of
generating capacity. Let us understand the meaning of this more
clearly, before moving on to the crucial question of how we shall
generate this power the world so desperately needs.
Thus, 1 kilowatt (1,000 watts) of
electricity, is equivalent to the work of about 1.33 muscular horses
of the working type. The horse cannot work all day, however, but
perhaps for only one third of it, after subtracting the time for
meals and rest. Thus, 1 kilowatt of electrical generating capacity,
available all day and night, could do the work of 3 times 1.33
horses, which equals 4 horses.
Without electricity, the work of those silent horses must be done by men and women, laboring to turn pumps, to carry water on their heads, to spend a whole day scrubbing clothes, and another heating irons on a fire to press them, while such simple requirements as water and sewage treatment, refrigeration, and even the light bulb, go wanting.
Such and worse remains the condition of
a majority of the world's population—some 1.7 billion people who are
entirely without electricity, and several billion more for whom the
supply is intermittent and deficient.
Thus, the amount available per person for use in China is less than 0.25 kilowatts, about one-third of a horsepower.
Taken over the full 24 hours, we can say
that the average person in China has available to him the work of 1
horse, compared to the 12 horses available in the United States. The
source of most U.S. manufactured products is the low-wage labor of
millions of Chinese, many of them from families with no access to
even the electric light.
They have never proven economically or technologically feasible, despite the enormous public expense in tax credits and subsidies which they have drawn upon.
Acciona’s Nevada Solar One concentrating solar power plant, the world’s largest, produces less than 15 megawatts of power, averaged over the course of a day.
To bring the present world population of 6.7 billion people up to a level of just 1.5 kilowatts of electrical generating capacity per capita will require that we build 6,000 gigawatts[2] (6 million megawatts) of generating capacity.
The only feasible way to accomplish this
is to embark now on a crash program to build nuclear power plants,
making use of our limited existing capabilities and gearing up for a
serial production capability for the new breed of fourth-generation,
high-temperature helium-cooled reactors, among other models.
The largest existing solar power plant, the solar concentrator known as Nevada Solar One, produces less than 15 megawatts of power, averaged over the course of the day.[3] The largest solar plant using photovoltaic panels is in Jumilla in southeastern Spain. It is rated at 23 megawatts maximum capacity.
Divide this by four, and you have the actual average output of less than 6 megawatts!
A single large nuclear power plant can produce 1,000 megawatts (1 gigawatt) or more of electrical power.
It can do this all day every day, not just when the Sun shines, and on a land surface area hundreds of times smaller than the equivalent solar plants or wind farms.
What Is Energy
Density?
A certain amount of work has to be done
to mine them and bring them to the place where they will be
consumed, but work also has to be done to utilize wind and solar, a
very great deal of work compared to the benefit received.
Let us examine the first term first, and see what we can learn from it.
Over the course of human history, there have been several progressive increases in the energy density of the fuels employed.
The transition from wood burning to coal
(which is almost four times more energy-dense than wood), took place
in Europe in the 18th Century. The higher temperatures and
regulation that could be achieved with coal fires permitted the
introduction of new technologies related to smelting of ores,
steelmaking, and other techniques. Until the 1950s, coal was the
primary energy source for industry and transportation, and it
remains the principal fuel used for electricity generation in the
U.S.A.
The advantage of oil over coal as a fuel for powering steam ships became a factor in geopolitics at the close of the 19th Century, with the conversion of the British Royal Navy from coal- to oil-fired steam boilers.
The weight advantage of oil, and its
ease of handling (not requiring manual stokers to feed the fire),
increased the range and efficiency of warships. The lighter
derivatives of petroleum, such as gasoline, benzene, and kerosene,
are among the most energy-dense liquids, which made them desirable
as a transportation fuel—as long as they last.
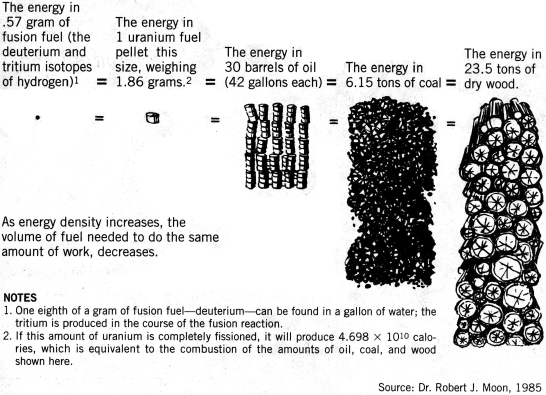
Fuel and Energy Comparisons
When compared by weight, the advantage of uranium fuel over the older types is as follows:
We shall be modest and note that these figures are derived by assuming that all of the fissionable uranium in the fuel pellet is burned up (fully fissioned).
The fuel burn-up rate in many presently operating reactors, may be only about 4%, although it is higher in advanced reactor designs. Thus, the figures above need to be divided by 25, giving nuclear power, in the worst-case scenario, an energy density advantage over wood, coal, and petroleum of only 88,000 to 460,000.
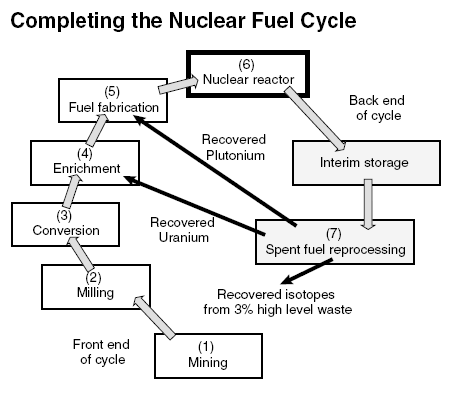
However, with fuel reprocessing, a form of recycling, the burn-up rate is nearly total. Because of the production of extra neutrons in the fission reaction, new fuel can be created by nuclear transmutation as the old fuel burns up.
The full nuclear fuel cycle, employing reprocessing and fuel breeding, is a virtually limitless cycle.
Nuclear is the only fuel that replaces itself as it burns.
It was one of the great achievements of 19th-Century physics, to demonstrate the equivalence of heat, electricity, and mechanical motion, resolving all these forms of energy (work), and others, to a common measure.
Thus, the technical definition of energy flux density would simply be the amount of energy passing across a given surface area in a unit of time. An example of a higher energy flux density could be had by comparing the capability of a sharp knife to a dull one. Holding the sharper knife, the same work exerted by the hand is concentrated over a smaller surface area.
The
energy flux density is greater and the sharp knife is able to cut
where the dull one cannot.
However, even this astounding numerical
advantage does not yet comprehend the essential difference. To
understand energy flux density in the context of physical economy, a
higher conception of work is required. It is not sufficient to
regard work, as we do in physics, merely as the expenditure of
energy measured in calories, joules, kilowatt-hours, or electron
volts.
Something akin to the skilled worker's maxim "don't work hard, work smart," is appropriate as a first approximation of the concept. Implied in the saying is the idea, that by application of the human mind, the same expenditure of effort can be made more efficient, perhaps by use of a different tool, or by the improvisation of a new one, or by organizing the process in a different way.
In the case of nuclear, as opposed to chemical or mechanical processes, a higher order sort of innovation is at work.
Here, we are dealing with the introduction of a new discovery of universal physical principle, the revolution in physical chemistry which began with the Curies' separation of the first gram of radium, and proceeded through the:
Apart from the questions of cost and efficiency, the fallacy of saying that wind and solar can be made to generate electricity, just as nuclear power can, is that it leaves out the transformative power which the application of this new universal physical principle permits.
Nuclear energy works smarter, vastly smarter, than wind,
solar, or fossil fuels ever can. The reason is not merely its
superior energy flux density, measured in caloric terms, but the
transformation in the physical economic process as a whole which it
can accomplish.
These particle/waves, which we call neutrons, have the ability to penetrate the nucleus of another nearby atom, and to transform it into a new element, a process known as transmutation. But this is only the beginning, for that new element may, in turn, spontaneously transmute into another, and another, producing a family of by-products (isotopes) which finally settle into a stable form.
By mastering the chemistry of these transformations, we have the ability to make new materials, some known and some yet to be discovered, which will be of benefit to future human life. We have also the benefit of the rays these isotopes give off, at least three different types, and each one at a different strength.
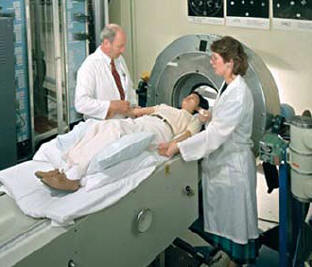
Their uses in diagnosis and treatment of an array of dangerous diseases are proven, and every day brings new possibilities.[7]
Ground wells are becoming contaminated as the fossil water supply within the ground becomes exhausted. Substantial regions of the United States, including Southern California and the American Southwest, are also reaching critical water supply limits.
Producing drinking water by desalination of seawater is a proven process. Currently, 40 million cubic meters of water a day are produced by desalination, mostly in the Middle East and North Africa. The leading methods are reverse osmosis, using electric-powered pumps to force salt or brackish water through a specially designed membrane, and flash distillation.
However, desalination is an energy-intensive process.
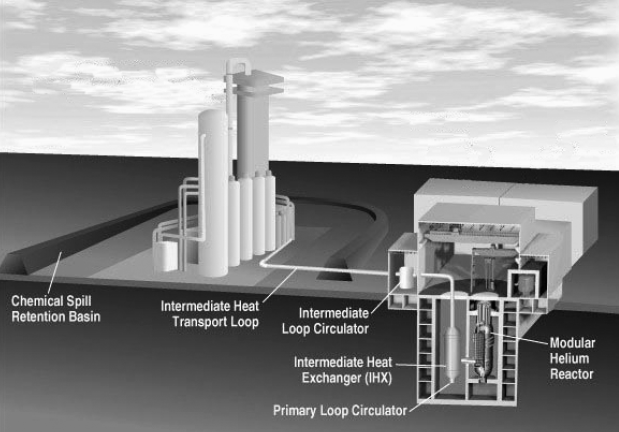
High-Temperature Reactor Coupled with Hydrogen Production Plant This General Atomics design for a high-temperature gas-cooled reactor couples its GT-MHR to a sulfur-iodine cycle hydrogen production plant. The sulfur-iodine cycle, which uses coupled chemical reactions and the heat from the high-temperature reactor, is the most promising thermochemical method for hydrogen production. Nuclear-produced hydrogen or hydrogen-based fuels in the future will provide the transportation fuels for the nation, replacing oil imports.
The feasibility of using nuclear power for large-scale desalination was first demonstrated nearly 40 years ago in Soviet Kazakhstan. For 27 years, the Aktau fast reactor produced 80,000 cubic meters per day of freshwater, and up to 135 megawatts of electric power at the same time.
Japan has operated ten demonstration desalination facilities linked to nuclear reactors, and India in 2002 set up a demonstration desalination plant at the Madras Atomic Power Station in the southeast, with a 6,300 cubic meter per day output.
Windmills and solar panels will not supply the large amounts
of electric power required to produce freshwater in dry areas of the
world, but nuclear plants can do it.
The key is the two atoms of hydrogen contained in every molecule of water. Hydrogen is a fuel, which can be utilized on its own, or combined with carbon sources to produce liquid fuels quite similar to those we now use. Hydrogen can be obtained from water either by electrolysis or by thermo-chemical splitting.
At the higher temperatures available from the new generation of modular helium-cooled reactors, the efficiency of both these processes is greatly increased.
Nuclear-produced hydrogen or
hydrogen-based fuels, combined with ample electricity for battery
vehicles, will provide a stable local supply of the transportation
fuel the nation needs. Instead of enriching the Anglo-Saudi oil
cartel by shipping petroleum across thousands of miles of ocean, we
can produce our own, cleaner fuel at domestic nuclear power plants,
while also providing our electricity and other needs.
Some breakthroughs, such as the practicable development of thermonuclear fusion energy, are almost now within our grasp. Others are yet to come.
To deny its application to our economy, and to return to 18th Century and earlier modes of power generation, is to stop human progress.
APPENDIX
Calculation of Energy in Electron Volts From
Burning a Fossil Fuel i (Example is methane, the principal component of natural gas)
The energy released in the fission of a single uranium atom is 200 million electron volts, making the simple advantage of uranium fission over combustion of natural gas about 20 million to 1.
However, the figure does not include the surface area over which the work occurs. In comparing nuclear to chemical reactions, we must consider the ratio of the surface area of the nucleus (about 10-24 cm2) to that of a molecule (about 10-15 cm2 for methane).
Thus an additional factor of 109 (1 billion) must be factored in, bringing the potential energy flux density advantage of nuclear fission over fossil fuel burning to approximately 20 quadrillion to 1.
This advantage is not yet realized in the present design of nuclear reactors, but demonstrates the potential still contained within this new regime of energy production.
References
|